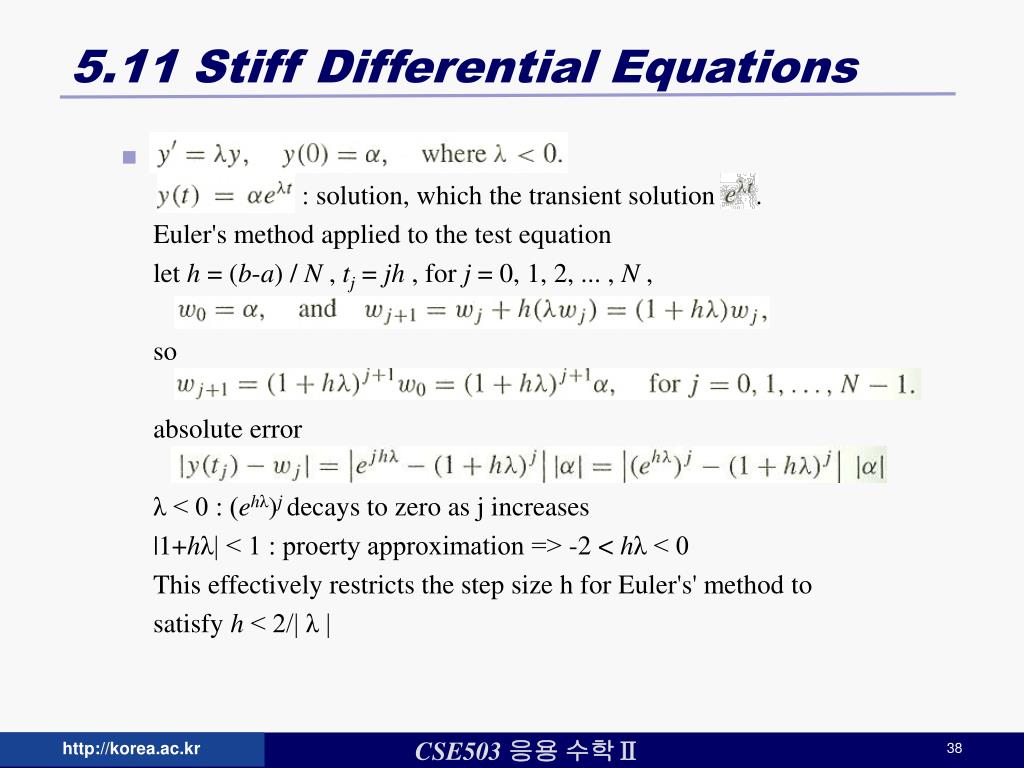
Stiff Differential Equation - The problem of stiffness leads to computational difficulty in. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods. 1) a stiff differential equation is numerically unstable unless the step size is extremely.
1) a stiff differential equation is numerically unstable unless the step size is extremely. The problem of stiffness leads to computational difficulty in. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods. 1) a stiff differential equation is numerically unstable unless the step size is extremely. The problem of stiffness leads to computational difficulty in.
stiffness and ordinary differential equation solving Jelena H. Pantel
1) a stiff differential equation is numerically unstable unless the step size is extremely. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods. The problem of stiffness leads to computational difficulty in.
Computational characteristics of feedforward neural networks for
1) a stiff differential equation is numerically unstable unless the step size is extremely. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. The problem of stiffness leads to computational difficulty in. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
Table 2 from A Sparse Differential Algebraic Equation (DAE) and Stiff
1) a stiff differential equation is numerically unstable unless the step size is extremely. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. The problem of stiffness leads to computational difficulty in. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
We numerically solve the differential Equation (35) for A = 0.2, and τ
Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods. The problem of stiffness leads to computational difficulty in. 1) a stiff differential equation is numerically unstable unless the step size is extremely.
Apostila Solve Stiff Differential Equations and DAEs Variable Order
1) a stiff differential equation is numerically unstable unless the step size is extremely. The problem of stiffness leads to computational difficulty in. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
What does a stiff differential equation mean? ResearchGate
The problem of stiffness leads to computational difficulty in. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. 1) a stiff differential equation is numerically unstable unless the step size is extremely. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
(PDF) Fresh approaches to the construction of parameterized neural
1) a stiff differential equation is numerically unstable unless the step size is extremely. The problem of stiffness leads to computational difficulty in. In mathematics, a stiff equation is a differential equation for which certain numerical methods. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >.
PPT Chapter 5. Ordinary Differential Equation PowerPoint Presentation
In mathematics, a stiff equation is a differential equation for which certain numerical methods. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. The problem of stiffness leads to computational difficulty in. 1) a stiff differential equation is numerically unstable unless the step size is extremely.
(PDF) A Sparse Differential Algebraic Equation (DAE) and Stiff Ordinary
In mathematics, a stiff equation is a differential equation for which certain numerical methods. 1) a stiff differential equation is numerically unstable unless the step size is extremely. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. The problem of stiffness leads to computational difficulty in.
Figure 3 from A Sparse Differential Algebraic Equation (DAE) and Stiff
1) a stiff differential equation is numerically unstable unless the step size is extremely. The problem of stiffness leads to computational difficulty in. Ordinary differential equations# given initial condition \(y_0 = y(t=0)\) , find \(y(t)\) for \(t >. In mathematics, a stiff equation is a differential equation for which certain numerical methods.
Ordinary Differential Equations# Given Initial Condition \(Y_0 = Y(T=0)\) , Find \(Y(T)\) For \(T >.
The problem of stiffness leads to computational difficulty in. In mathematics, a stiff equation is a differential equation for which certain numerical methods. 1) a stiff differential equation is numerically unstable unless the step size is extremely.