Laplacian Differential Equation - The laplace equation is a basic pde that arises in the heat and diffusion equations. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. Laplace’s partial differential equation in two dimensions: In this section we discuss solving laplace’s equation. As we will see this is. Laplace's equation and harmonic functions in this section, we will show how green's theorem.
The laplace equation is a basic pde that arises in the heat and diffusion equations. Laplace's equation and harmonic functions in this section, we will show how green's theorem. In this section we discuss solving laplace’s equation. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. As we will see this is. Laplace’s partial differential equation in two dimensions:
Laplace’s partial differential equation in two dimensions: The laplace equation is a basic pde that arises in the heat and diffusion equations. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. In this section we discuss solving laplace’s equation. Laplace's equation and harmonic functions in this section, we will show how green's theorem. As we will see this is.
(PDF) On SecondOrder Differential Equations with Nonhomogeneous Φ
Laplace's equation and harmonic functions in this section, we will show how green's theorem. Laplace’s partial differential equation in two dimensions: The laplace equation is a basic pde that arises in the heat and diffusion equations. In this section we discuss solving laplace’s equation. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where.
(PDF) Iterative Solutions for the Differential Equation with Laplacian
The laplace equation is a basic pde that arises in the heat and diffusion equations. Laplace’s partial differential equation in two dimensions: In this section we discuss solving laplace’s equation. Laplace's equation and harmonic functions in this section, we will show how green's theorem. As we will see this is.
(PDF) Periodic solutions for a Liénard type pLaplacian differential
The laplace equation is a basic pde that arises in the heat and diffusion equations. As we will see this is. In this section we discuss solving laplace’s equation. Laplace's equation and harmonic functions in this section, we will show how green's theorem. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where.
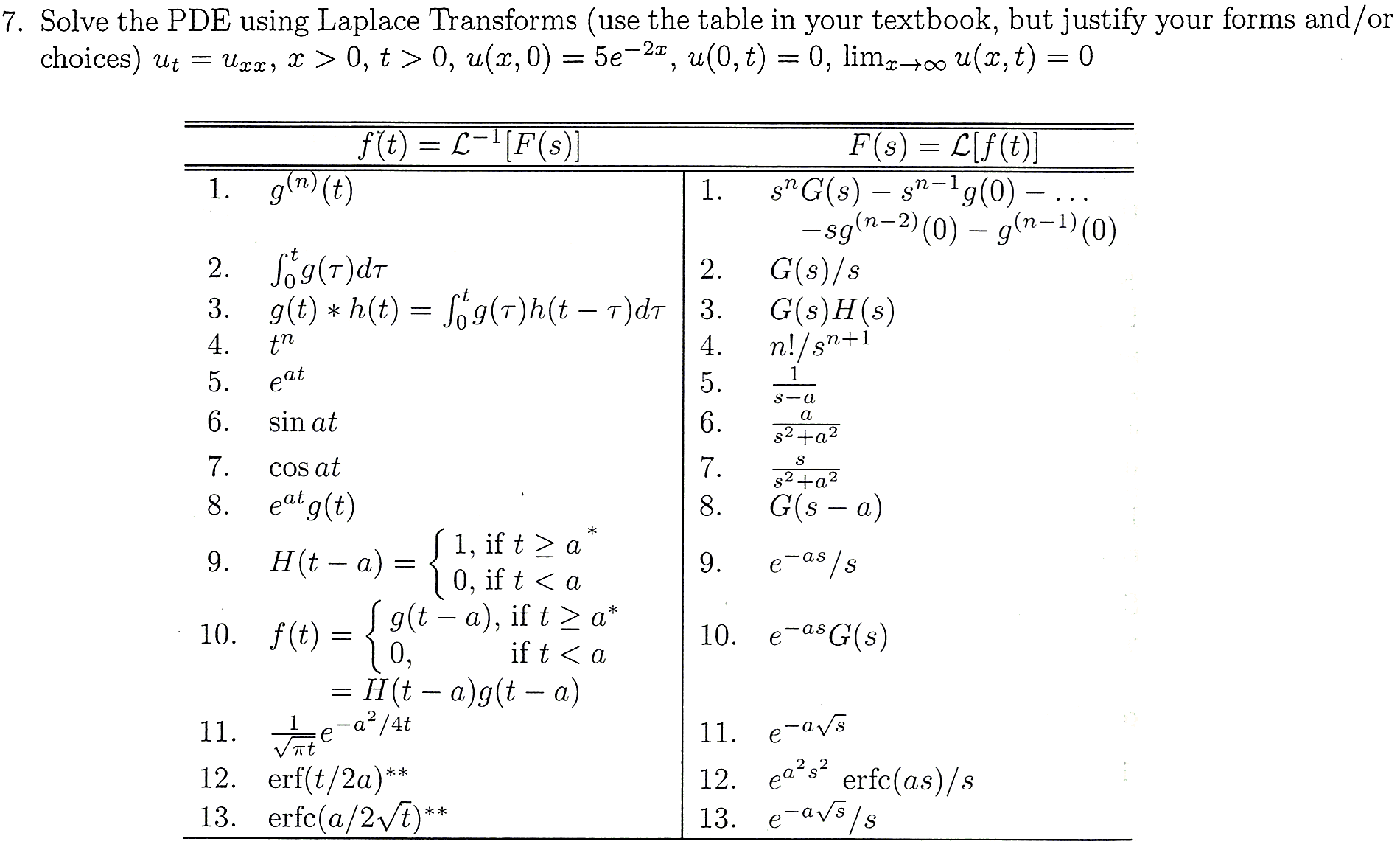
Solved 7. Solve the Partial Differential Equation using
The laplace equation is a basic pde that arises in the heat and diffusion equations. As we will see this is. In this section we discuss solving laplace’s equation. Laplace's equation and harmonic functions in this section, we will show how green's theorem. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where.
(PDF) Solving the Laplacian Equation in 3D using Finite Element Method
Laplace's equation and harmonic functions in this section, we will show how green's theorem. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. Laplace’s partial differential equation in two dimensions: The laplace equation is a basic pde that arises in the heat and diffusion equations. In this section we discuss solving laplace’s equation.
Laplacian Smoothing PerTriangle Values
Laplace’s partial differential equation in two dimensions: As we will see this is. In this section we discuss solving laplace’s equation. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. The laplace equation is a basic pde that arises in the heat and diffusion equations.
The Laplacian in Curvilinear Coordinates The Full Story PDF PDF
Laplace's equation and harmonic functions in this section, we will show how green's theorem. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. As we will see this is. In this section we discuss solving laplace’s equation. The laplace equation is a basic pde that arises in the heat and diffusion equations.
differential geometry Prove that Laplacian is selfadjoint
(1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. In this section we discuss solving laplace’s equation. The laplace equation is a basic pde that arises in the heat and diffusion equations. Laplace's equation and harmonic functions in this section, we will show how green's theorem. Laplace’s partial differential equation in two dimensions:
SPECTRAL GEOMETRY OF THE LAPLACIAN SPECTRAL ANALYSIS
In this section we discuss solving laplace’s equation. Laplace's equation and harmonic functions in this section, we will show how green's theorem. The laplace equation is a basic pde that arises in the heat and diffusion equations. (1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. Laplace’s partial differential equation in two dimensions:
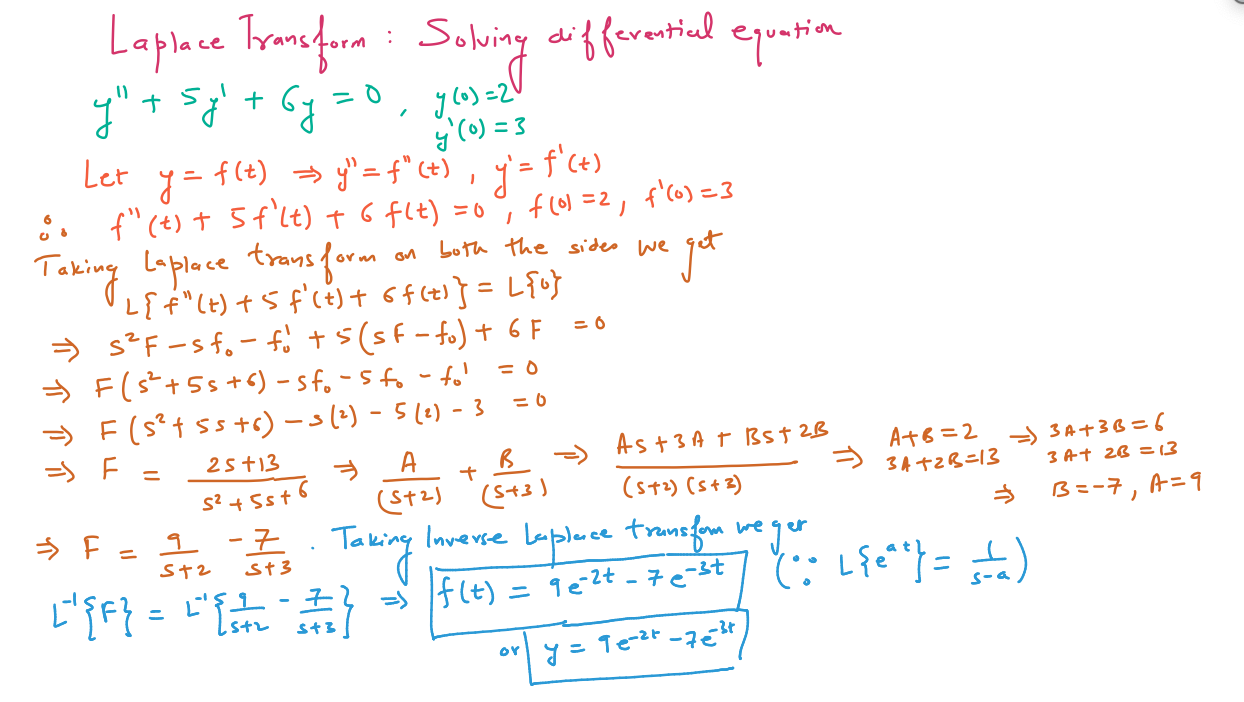
Laplace Transform Solving Differential Equation Sumant's 1 page of Math
(1) ∂2w ∂x2 + ∂2w ∂y2 = 0, where. The laplace equation is a basic pde that arises in the heat and diffusion equations. As we will see this is. In this section we discuss solving laplace’s equation. Laplace’s partial differential equation in two dimensions:
(1) ∂2W ∂X2 + ∂2W ∂Y2 = 0, Where.
As we will see this is. The laplace equation is a basic pde that arises in the heat and diffusion equations. Laplace’s partial differential equation in two dimensions: Laplace's equation and harmonic functions in this section, we will show how green's theorem.