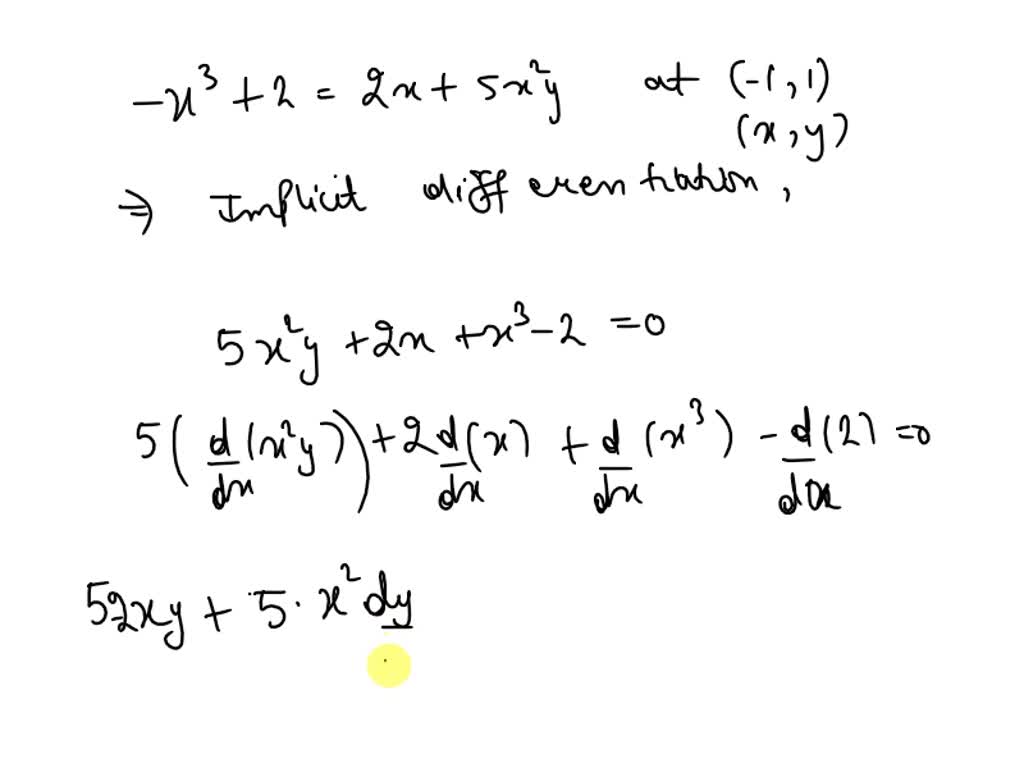Implicit Differentiation With Three Variables - If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. Now, we did this problem because implicit differentiation works in exactly the. In this section we will discuss implicit differentiation. How do i take the implicit partial derivative when the variable is not equal to the equation? Perform implicit differentiation of a function of two or more variables. In this unit we explain how these can be differentiated using implicit differentiation.
In this unit we explain how these can be differentiated using implicit differentiation. How do i take the implicit partial derivative when the variable is not equal to the equation? If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. Perform implicit differentiation of a function of two or more variables. Now, we did this problem because implicit differentiation works in exactly the. In this section we will discuss implicit differentiation.
How do i take the implicit partial derivative when the variable is not equal to the equation? Perform implicit differentiation of a function of two or more variables. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. In this unit we explain how these can be differentiated using implicit differentiation. In this section we will discuss implicit differentiation. Now, we did this problem because implicit differentiation works in exactly the.
3.7 Implicit Differentiation
In this section we will discuss implicit differentiation. Perform implicit differentiation of a function of two or more variables. How do i take the implicit partial derivative when the variable is not equal to the equation? If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. In this unit we explain how.
Implicit Differentiation PDF Mathematical Analysis Differential
Now, we did this problem because implicit differentiation works in exactly the. How do i take the implicit partial derivative when the variable is not equal to the equation? Perform implicit differentiation of a function of two or more variables. In this unit we explain how these can be differentiated using implicit differentiation. In this section we will discuss implicit.
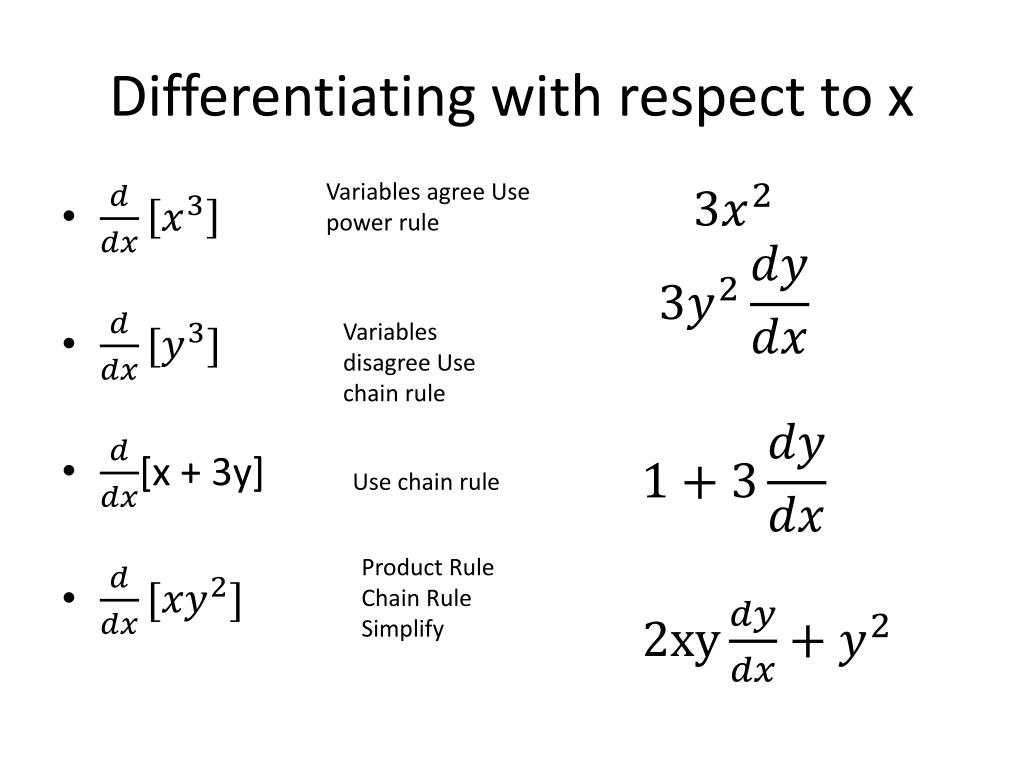
PPT Implicit Differentiation PowerPoint Presentation, free download
In this section we will discuss implicit differentiation. Now, we did this problem because implicit differentiation works in exactly the. Perform implicit differentiation of a function of two or more variables. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. How do i take the implicit partial derivative when the variable.
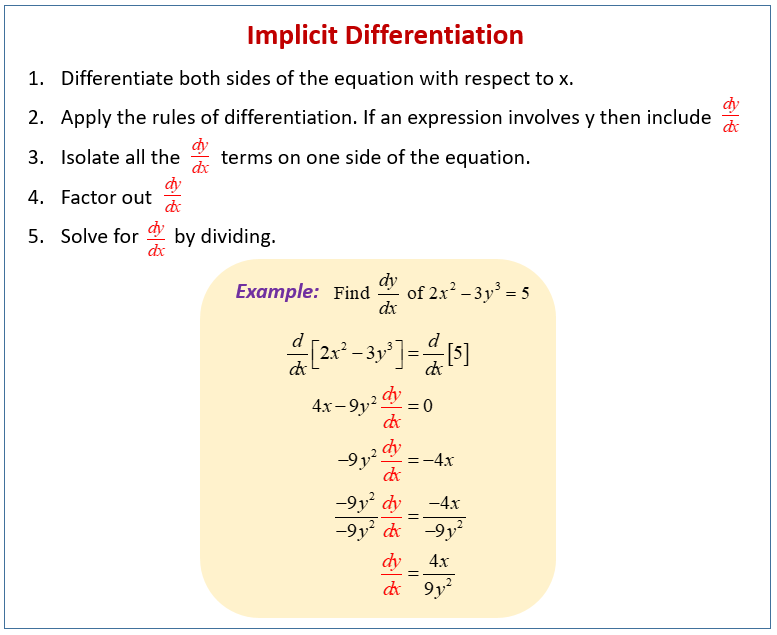
How to Do Implicit Differentiation 7 Steps (with Pictures)
Perform implicit differentiation of a function of two or more variables. How do i take the implicit partial derivative when the variable is not equal to the equation? In this unit we explain how these can be differentiated using implicit differentiation. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. Now,.
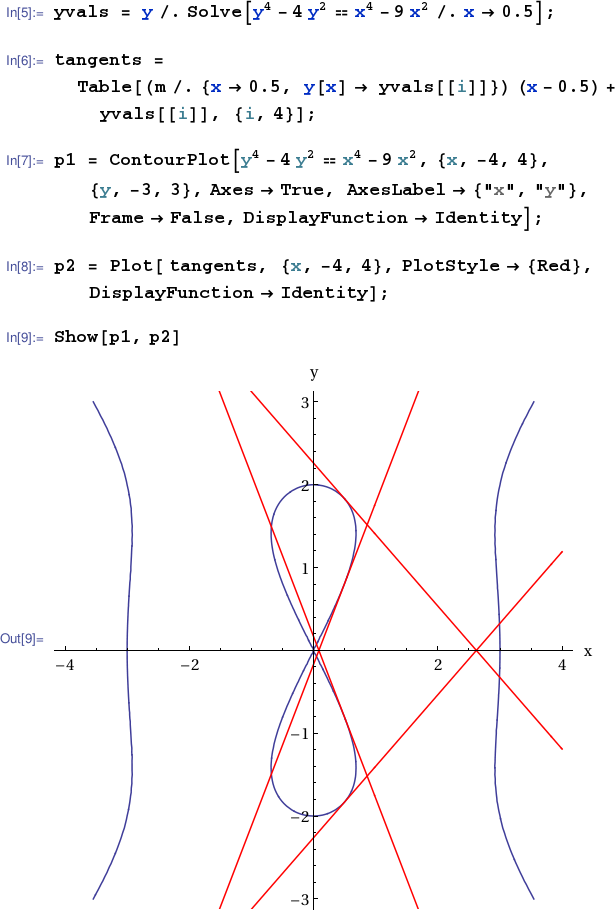
Implicit Differentiation Examples Implicit Derivative
How do i take the implicit partial derivative when the variable is not equal to the equation? In this unit we explain how these can be differentiated using implicit differentiation. In this section we will discuss implicit differentiation. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. Now, we did this.
calculus Implicit Partial Differentiation Mathematics Stack Exchange
Now, we did this problem because implicit differentiation works in exactly the. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. In this unit we explain how these can be differentiated using implicit differentiation. In this section we will discuss implicit differentiation. How do i take the implicit partial derivative when.
intro to Implicit differentiation PDF Free Download
In this section we will discuss implicit differentiation. Now, we did this problem because implicit differentiation works in exactly the. How do i take the implicit partial derivative when the variable is not equal to the equation? If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. Perform implicit differentiation of a.
3.7 Implicit Differentiation
In this unit we explain how these can be differentiated using implicit differentiation. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. In this section we will discuss implicit differentiation. Perform implicit differentiation of a function of two or more variables. How do i take the implicit partial derivative when the.
SOLVED Implicit differentiation examples
Now, we did this problem because implicit differentiation works in exactly the. How do i take the implicit partial derivative when the variable is not equal to the equation? In this unit we explain how these can be differentiated using implicit differentiation. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f..
Examples using Implicit Differentiation (solutions, formulas, videos)
Now, we did this problem because implicit differentiation works in exactly the. Perform implicit differentiation of a function of two or more variables. If the equation x2 +y2 +z2 = g(ax + by + cz) defines z = f(x, y), f. How do i take the implicit partial derivative when the variable is not equal to the equation? In this.
If The Equation X2 +Y2 +Z2 = G(Ax + By + Cz) Defines Z = F(X, Y), F.
In this section we will discuss implicit differentiation. Now, we did this problem because implicit differentiation works in exactly the. Perform implicit differentiation of a function of two or more variables. In this unit we explain how these can be differentiated using implicit differentiation.