Implicit Differentiation Of Trig Functions - In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. Use the chain rule to differentiate composite functions. In this section we will discuss differentiating trig functions. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Obtain and prove a formula for its derivative directly from the definition of derivative as a. Here is a set of practice problems to accompany the implicit differentiation.
In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. In this section we will discuss differentiating trig functions. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. Obtain and prove a formula for its derivative directly from the definition of derivative as a. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Use the chain rule to differentiate composite functions. Here is a set of practice problems to accompany the implicit differentiation.
In this section we will discuss differentiating trig functions. Obtain and prove a formula for its derivative directly from the definition of derivative as a. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Here is a set of practice problems to accompany the implicit differentiation. In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. Use the chain rule to differentiate composite functions.
Implicit Differentiation (w/ Examples And Worksheets!)
Here is a set of practice problems to accompany the implicit differentiation. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. In the following video, we use this trick to differentiate the inverse trig.
Implicit Differentiation (w/ Examples And Worksheets!)
First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. In this section we will discuss differentiating trig functions. Here.
Implicit Differentiation Practice Quiz
Obtain and prove a formula for its derivative directly from the definition of derivative as a. In this section we will discuss differentiating trig functions. In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of.
Implicit Differentiation and Inverse Trig Functions NoraCuranaj
For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Obtain and prove a formula for its derivative directly from the definition of derivative as a. Here is a set of practice problems to accompany.
Implicit Differentiation Calculus Study guide
In this section we will discuss differentiating trig functions. Obtain and prove a formula for its derivative directly from the definition of derivative as a. Use the chain rule to differentiate composite functions. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative.
ShowMe differentiation trig
First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. In this section we will discuss differentiating trig functions. Here is a set of practice problems to accompany the implicit differentiation. Use the chain rule to differentiate composite functions.
Implicit Differentiation Inverse Trig Math 115, Implicit
In the following video, we use this trick to differentiate the inverse trig functions $\sin^{. Obtain and prove a formula for its derivative directly from the definition of derivative as a. Use the chain rule to differentiate composite functions. In this section we will discuss differentiating trig functions. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$.
Implicit Differentiation Example on Trigonometry PeakD
Here is a set of practice problems to accompany the implicit differentiation. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. In this section we will discuss differentiating trig functions. Obtain and prove a formula for its derivative directly from the definition.
Trig Identities Derivatives [Inverse, Antiderivative]
Use the chain rule to differentiate composite functions. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Here is a set of practice problems to accompany the implicit differentiation. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative of y − 2x y − 2 x. In this section we will.
Implicit Differentiation W Examples And Worksheets 25000 Hot Sex Picture
Obtain and prove a formula for its derivative directly from the definition of derivative as a. In this section we will discuss differentiating trig functions. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$. Use the chain rule to differentiate composite functions. For the chain rule, you want to multiply cos(y − 2x) cos (y − 2 x) by the derivative.
Use The Chain Rule To Differentiate Composite Functions.
In this section we will discuss differentiating trig functions. Here is a set of practice problems to accompany the implicit differentiation. Obtain and prove a formula for its derivative directly from the definition of derivative as a. First, you should be writing $\frac{d}{dx}$, not $\frac{dy}{dx}$.
For The Chain Rule, You Want To Multiply Cos(Y − 2X) Cos (Y − 2 X) By The Derivative Of Y − 2X Y − 2 X.
In the following video, we use this trick to differentiate the inverse trig functions $\sin^{.
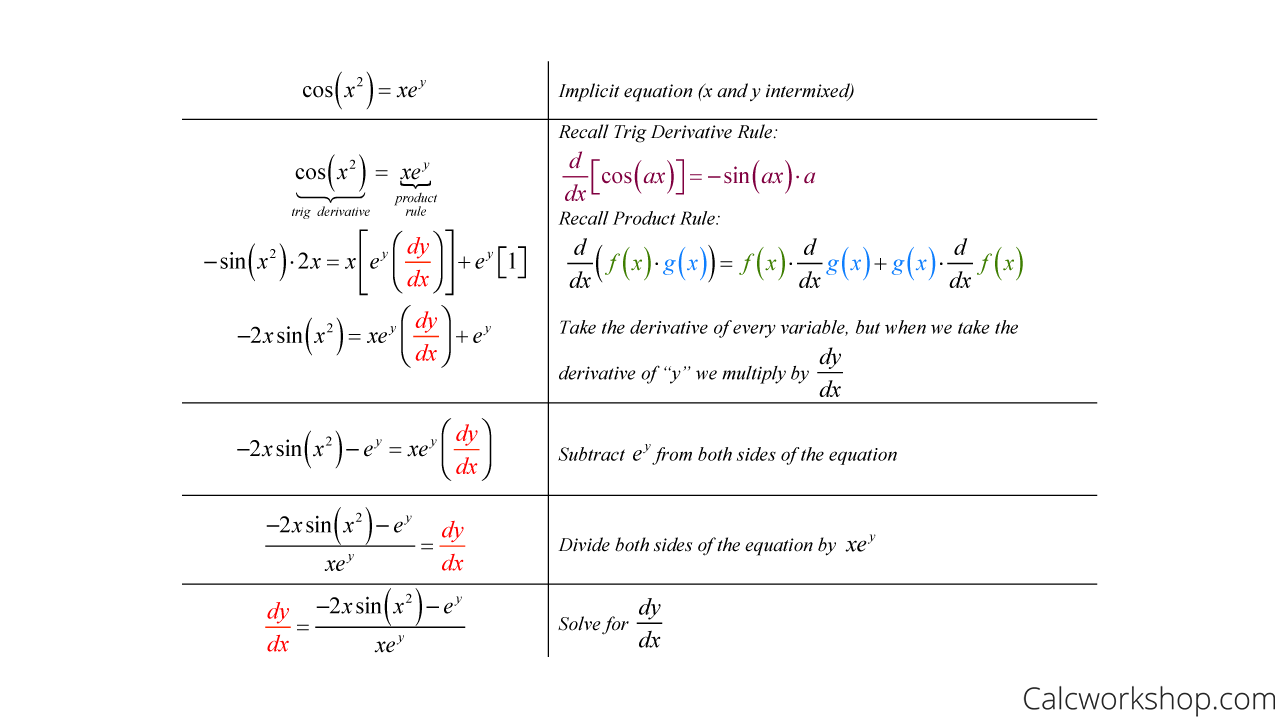
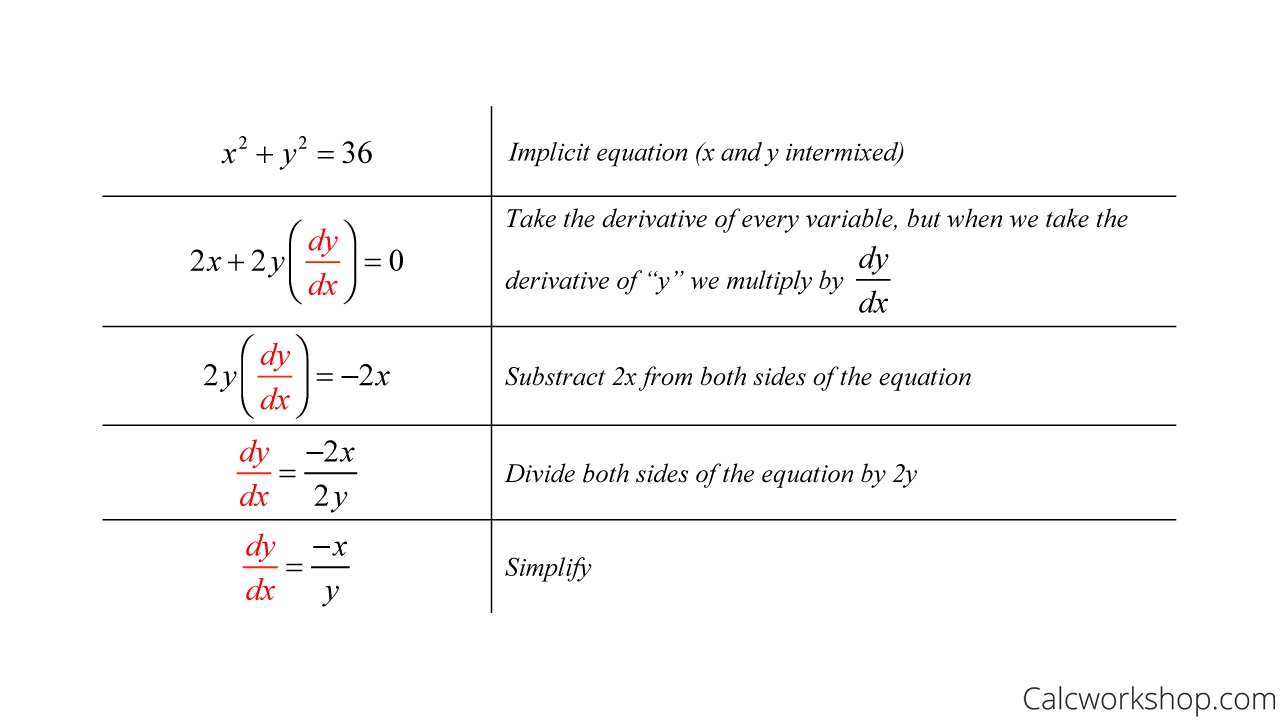





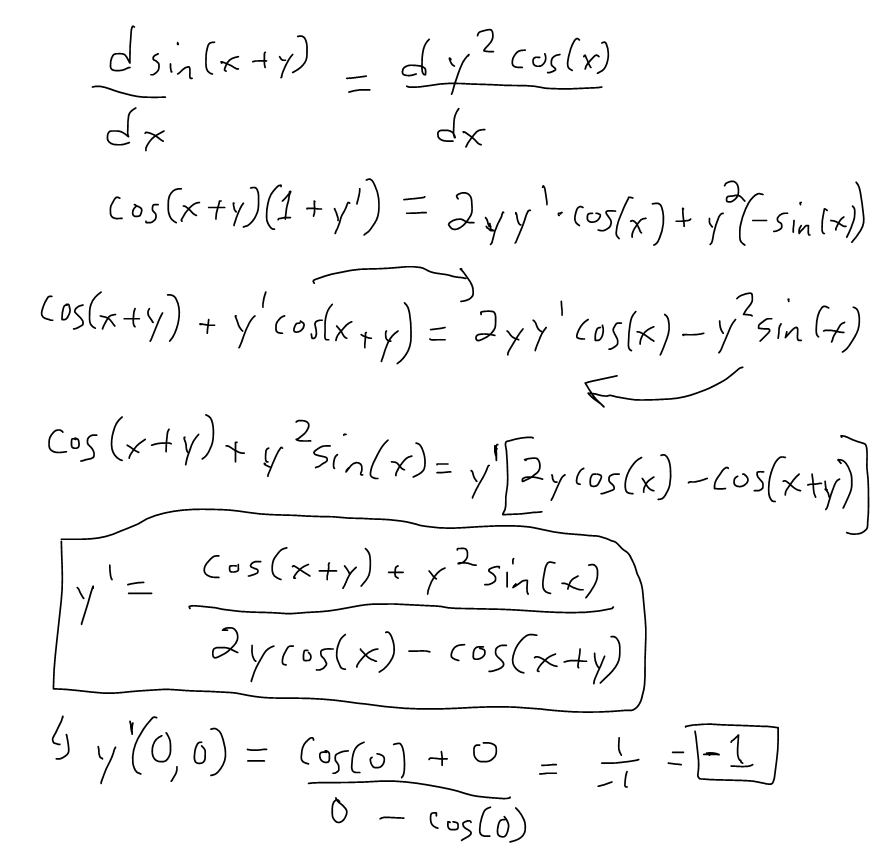
![Trig Identities Derivatives [Inverse, Antiderivative]](https://trigidentities.net/wp-content/uploads/2022/10/Inverse-Trig-Identities-Derivatives-1024x888.jpg)
