Implicit Differentiation Multivariable - How to find partial derivatives of an implicitly defined multivariable function using the implicit. So, basically what we’re doing here is differentiating \ (f\) with respect to each. Learn how to compute partial derivatives of variables related by an equation of the form f. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. Perform implicit differentiation of a function of two or more variables.
The multivariable chain rule allows us to compute implicit derivatives easily by just computing. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. So, basically what we’re doing here is differentiating \ (f\) with respect to each. Learn how to compute partial derivatives of variables related by an equation of the form f. How to find partial derivatives of an implicitly defined multivariable function using the implicit. Perform implicit differentiation of a function of two or more variables.
How to find partial derivatives of an implicitly defined multivariable function using the implicit. Perform implicit differentiation of a function of two or more variables. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. So, basically what we’re doing here is differentiating \ (f\) with respect to each. Learn how to compute partial derivatives of variables related by an equation of the form f. The multivariable chain rule allows us to compute implicit derivatives easily by just computing.
SOLUTION Partial Differentiation Surface in Implicit Form A geometric
Learn how to compute partial derivatives of variables related by an equation of the form f. Perform implicit differentiation of a function of two or more variables. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. So, basically what we’re doing here is differentiating \ (f\) with respect to each. How to.
How to Do Implicit Differentiation 7 Steps (with Pictures)
Perform implicit differentiation of a function of two or more variables. Learn how to compute partial derivatives of variables related by an equation of the form f. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. How to find partial derivatives of an implicitly defined multivariable function using the implicit. If the equation $f(x,y,z)=0$ defines.
SOLUTION Partial Differentiation Surface in Implicit Form A geometric
Perform implicit differentiation of a function of two or more variables. So, basically what we’re doing here is differentiating \ (f\) with respect to each. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. How to find partial derivatives of an implicitly defined multivariable function using the implicit. Learn how to compute.
Implicit Differentiation Examples Youtube
So, basically what we’re doing here is differentiating \ (f\) with respect to each. Perform implicit differentiation of a function of two or more variables. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. Learn how to compute partial derivatives of variables related by an equation of the form f. If the equation $f(x,y,z)=0$ defines.
calculus Implicit Partial Differentiation Mathematics Stack Exchange
So, basically what we’re doing here is differentiating \ (f\) with respect to each. Learn how to compute partial derivatives of variables related by an equation of the form f. How to find partial derivatives of an implicitly defined multivariable function using the implicit. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then.
Implicit Differentiation (w/ Examples And Worksheets!)
Learn how to compute partial derivatives of variables related by an equation of the form f. How to find partial derivatives of an implicitly defined multivariable function using the implicit. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then.
intro to Implicit differentiation PDF Free Download
If the equation $f(x,y,z)=0$ defines $z$ implicitly as a differentiable function of x and y, then by. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. Learn how to compute partial derivatives of variables related by an equation of the form f. How to find partial derivatives of an implicitly defined multivariable function using the.
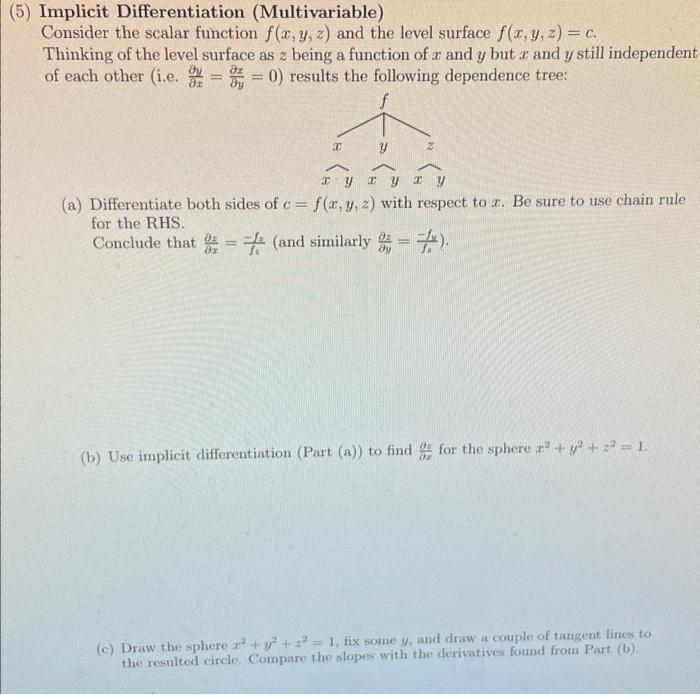
Solved Implicit Differentiation (Multivariable) Consider the
Learn how to compute partial derivatives of variables related by an equation of the form f. Perform implicit differentiation of a function of two or more variables. So, basically what we’re doing here is differentiating \ (f\) with respect to each. How to find partial derivatives of an implicitly defined multivariable function using the implicit. The multivariable chain rule allows.
How to Do Implicit Differentiation 7 Steps (with Pictures)
Perform implicit differentiation of a function of two or more variables. How to find partial derivatives of an implicitly defined multivariable function using the implicit. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. So, basically what we’re doing here is differentiating \ (f\) with respect to each. Learn how to compute partial derivatives of.
Chain rule & implicit differentiation Multivariable Calculus Studocu
Perform implicit differentiation of a function of two or more variables. How to find partial derivatives of an implicitly defined multivariable function using the implicit. Learn how to compute partial derivatives of variables related by an equation of the form f. So, basically what we’re doing here is differentiating \ (f\) with respect to each. The multivariable chain rule allows.
If The Equation $F(X,Y,Z)=0$ Defines $Z$ Implicitly As A Differentiable Function Of X And Y, Then By.
Learn how to compute partial derivatives of variables related by an equation of the form f. So, basically what we’re doing here is differentiating \ (f\) with respect to each. The multivariable chain rule allows us to compute implicit derivatives easily by just computing. How to find partial derivatives of an implicitly defined multivariable function using the implicit.