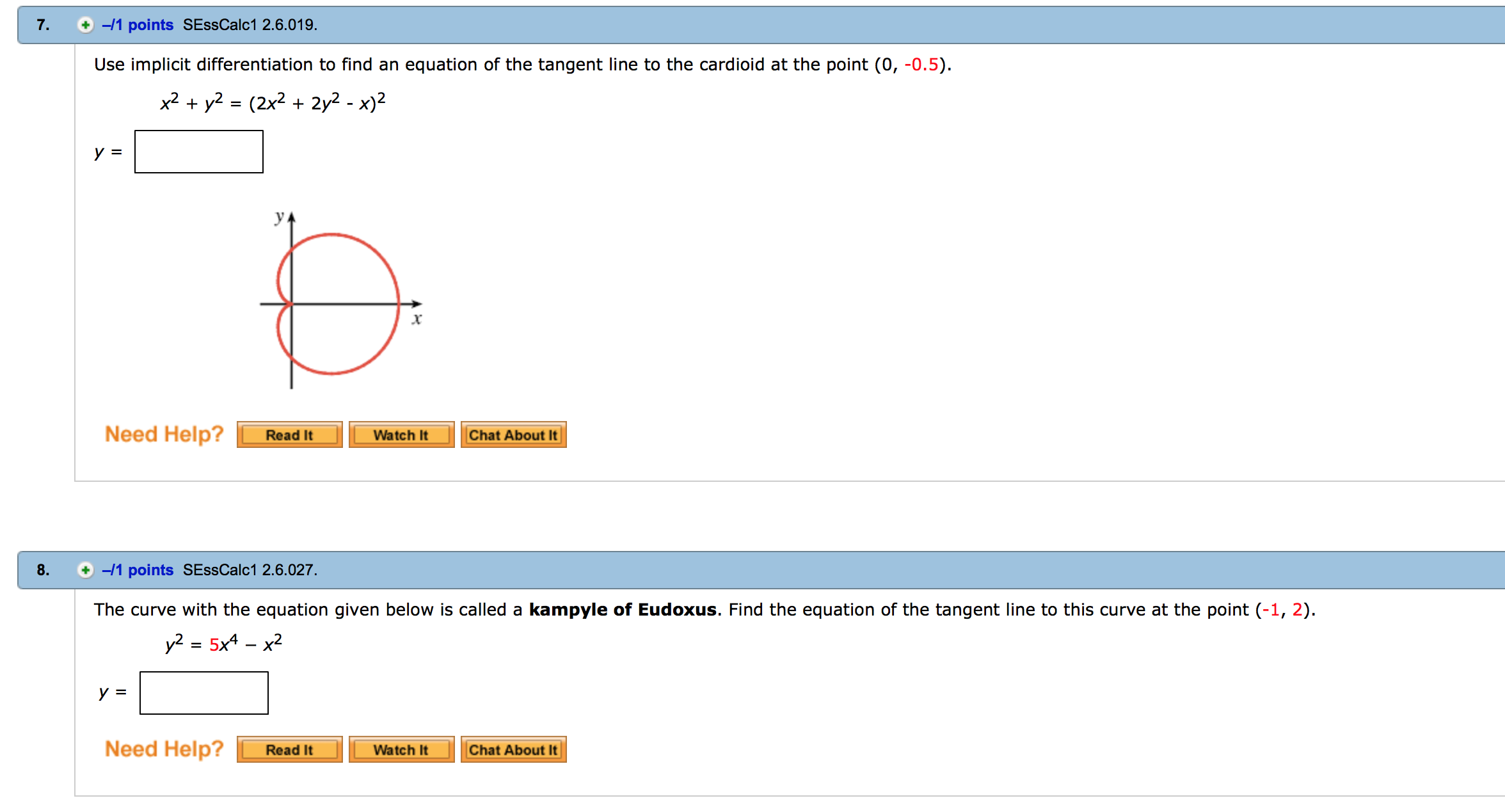
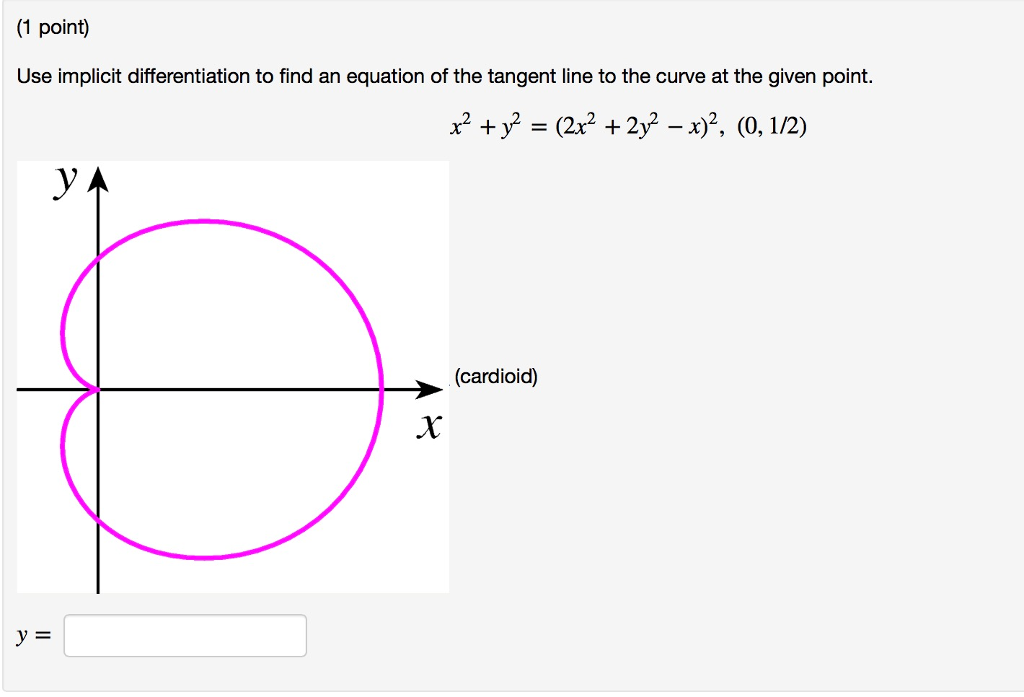
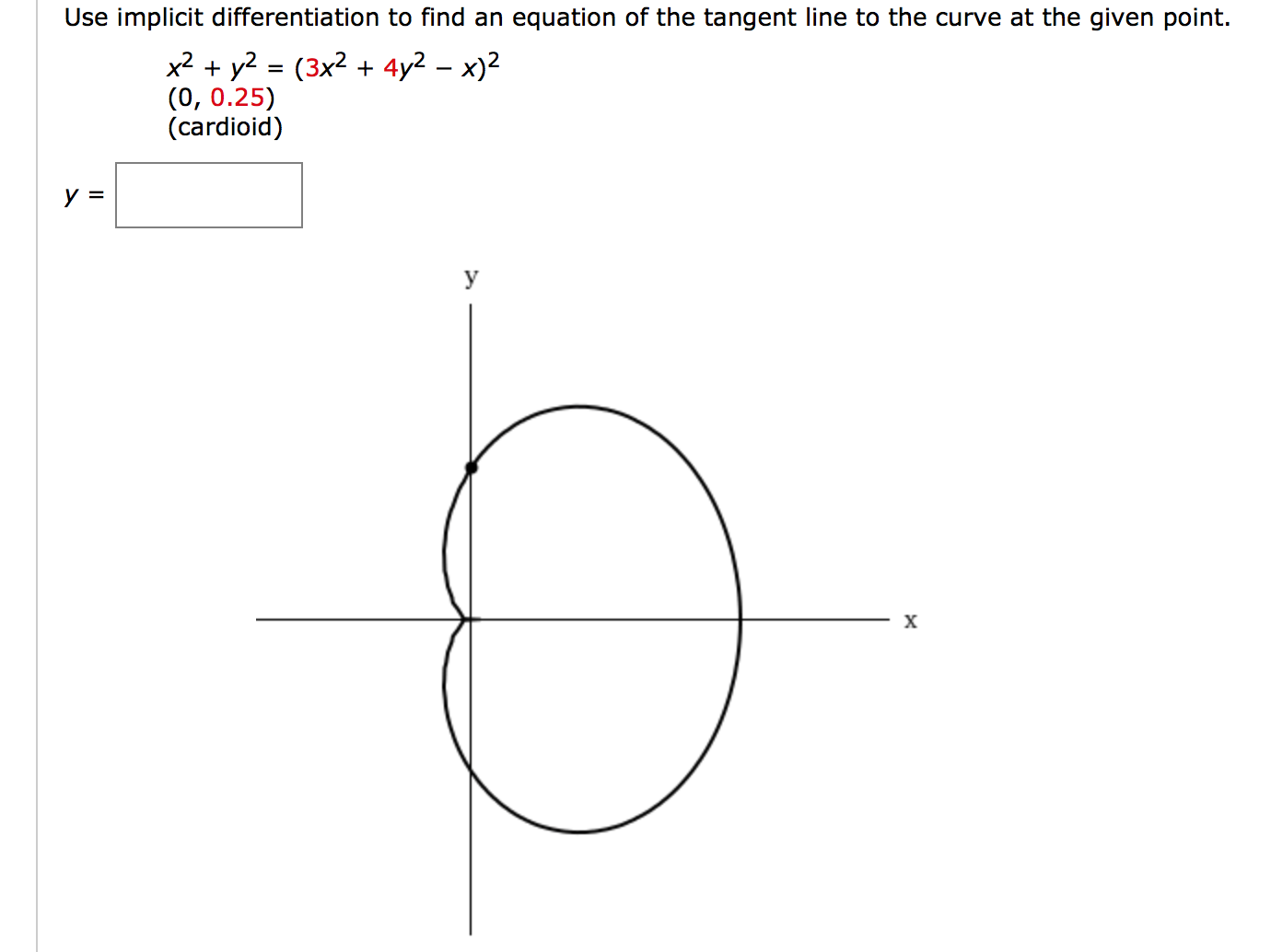
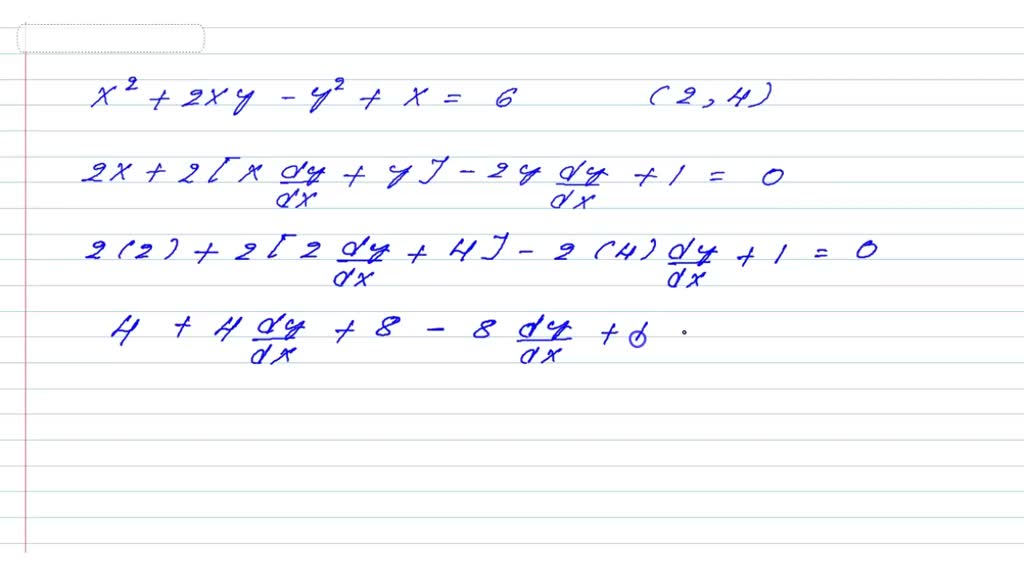How To Find Vertical Tangent Line Implicit Differentiation - Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ The typical way to get used to implicit. In this article, we’ll show you how to find vertical and horizontal tangent lines using implicit differentiation. We find the first derivative and then consider the. The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. Finding the vertical and horizontal tangent lines to an implicitly defined curve.
The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. The typical way to get used to implicit. We find the first derivative and then consider the. Finding the vertical and horizontal tangent lines to an implicitly defined curve. Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. In this article, we’ll show you how to find vertical and horizontal tangent lines using implicit differentiation.
Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ Finding the vertical and horizontal tangent lines to an implicitly defined curve. Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. The typical way to get used to implicit. In this article, we’ll show you how to find vertical and horizontal tangent lines using implicit differentiation. The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. We find the first derivative and then consider the.
Solved Use Implicit Differentiation To Find An Equation O...
In this article, we’ll show you how to find vertical and horizontal tangent lines using implicit differentiation. Finding the vertical and horizontal tangent lines to an implicitly defined curve. Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ The typical way to get used to implicit. The graph of z1.
Solved Use implicit differentiation to find an equation of
The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. The typical.
SOLVED Use implicit differentiation to find an equation of the tangent
Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ The typical way to get used to implicit. We find the first derivative and then consider the. Finding the vertical and horizontal tangent lines to an implicitly defined curve. In this article, we’ll show you how to find vertical and horizontal.
Solved Use implicit differentiation to find an equation of
Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve for f.
SOLVED point) Use implicit differentiation to find an equation of the
Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. The typical way to get used to implicit. The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0,.
Answered Use implicit differentiation to find an… bartleby
Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. Finding the vertical and horizontal tangent lines to an implicitly defined curve. We find the first derivative and then consider the. The graph of z1 shown in lesson 13.1.
Answered Use implicit differentiation to find an… bartleby
The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. We find.
Solved Use implicit differentiation to find an equation of
In this article, we’ll show you how to find vertical and horizontal tangent lines using implicit differentiation. The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Finding the vertical and horizontal tangent lines to an implicitly defined curve. Ef (x) f ′.
Solved Use implicit differentiation to find an equation of
The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve for f ′ (x) f ′ (x) = e − f (x) = e − logx = 1 x. In this.
Answered Use implicit differentiation to find an… bartleby
Finding the vertical and horizontal tangent lines to an implicitly defined curve. We find the first derivative and then consider the. The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a. Ef (x) f ′ (x) = 1 and we can now solve.
Ef (X) F ′ (X) = 1 And We Can Now Solve For F ′ (X) F ′ (X) = E − F (X) = E − Logx = 1 X.
Finding the vertical and horizontal tangent lines to an implicitly defined curve. Use implicit differentiation to find an equation of the tangent line to the curve at the given point $(2,4)$ We find the first derivative and then consider the. The typical way to get used to implicit.
In This Article, We’ll Show You How To Find Vertical And Horizontal Tangent Lines Using Implicit Differentiation.
The graph of z1 shown in lesson 13.1 suggests that one branch of the curve has a horizontal tangent at (0, 0) and another branch has a.