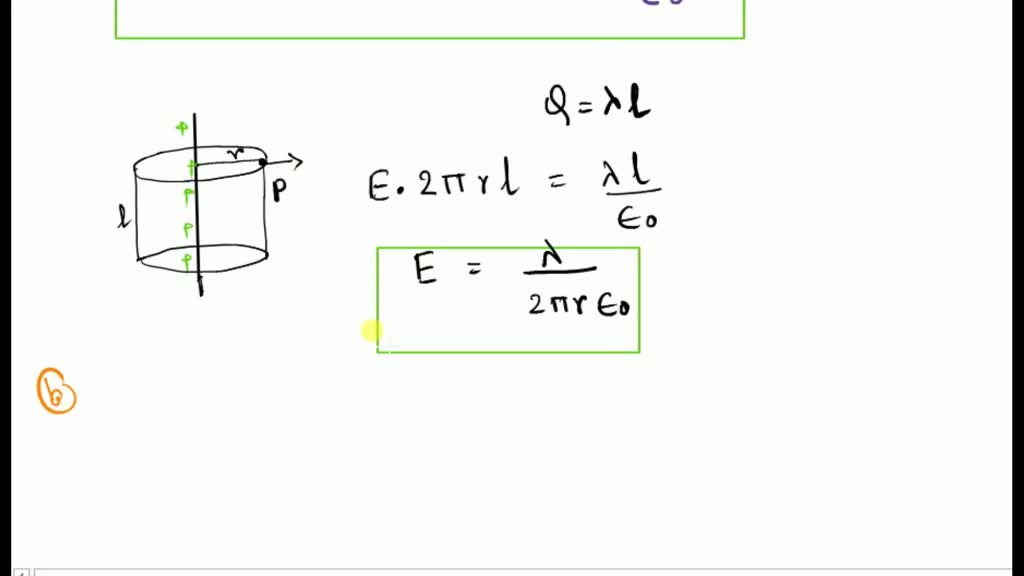
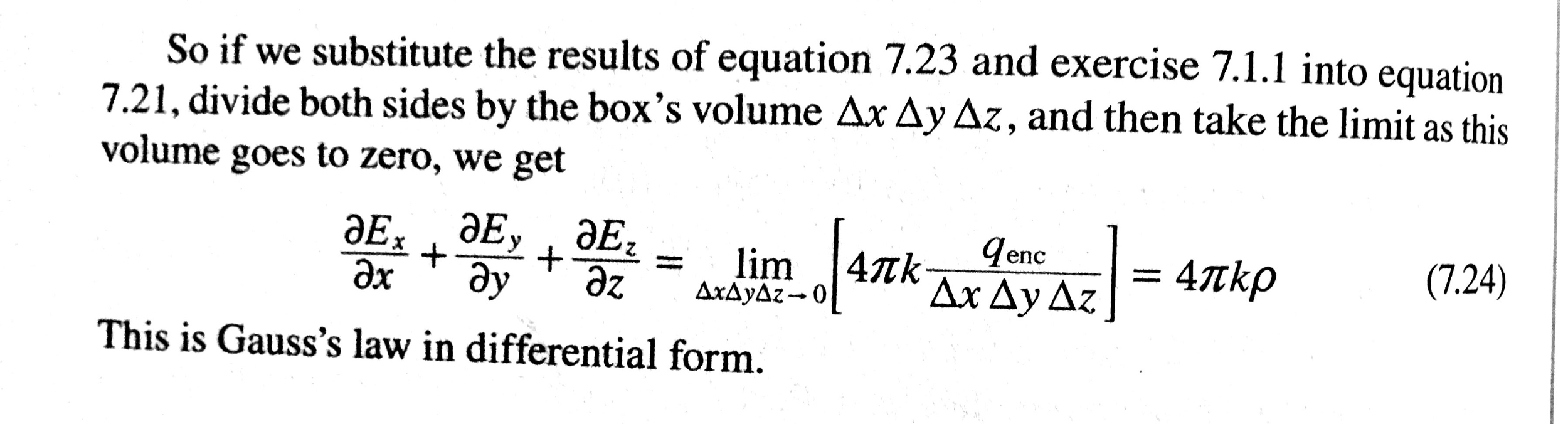Gauss Law Differential Form - This conclusion is the differential form of gauss' law, and is one of maxwell's equations. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. It states that the divergence of the electric field at any. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field.
(1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. It states that the divergence of the electric field at any. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space.
(1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. It states that the divergence of the electric field at any. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. This conclusion is the differential form of gauss' law, and is one of maxwell's equations.
Solved Using the differential form of Gauss's law, determine
Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. The differential form of gauss law.
SOLUTION Integral and differential form of gauss s law Studypool
Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. It states that the divergence of the electric field at any. The differential form of gauss law relates the electric field to the charge distribution.
Solved BOX 7.1 Gauss's Law in Integral and Differential Form
Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. The differential form of gauss law.
electrostatics Problem in understanding Differential form of Gauss's
Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. It states that the divergence of the electric field at any. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. The differential form of gauss law relates the electric field to the charge distribution.
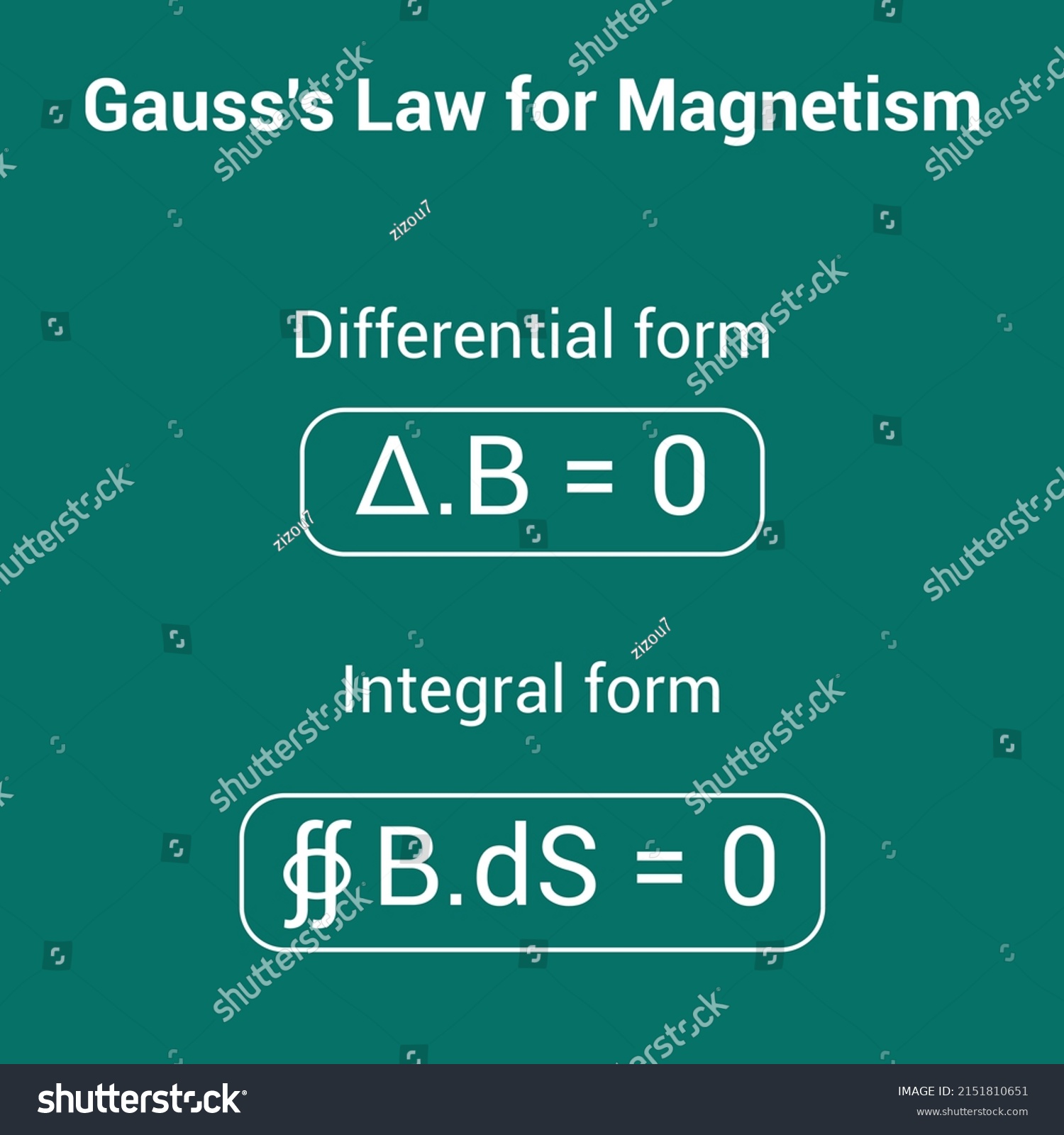
Differential Integral Form Gauss Law Stock Vector (Royalty
Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. This.
Solved 1. Gauss' law in differential form involves the
(1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. Gauss' law in differential form lends.
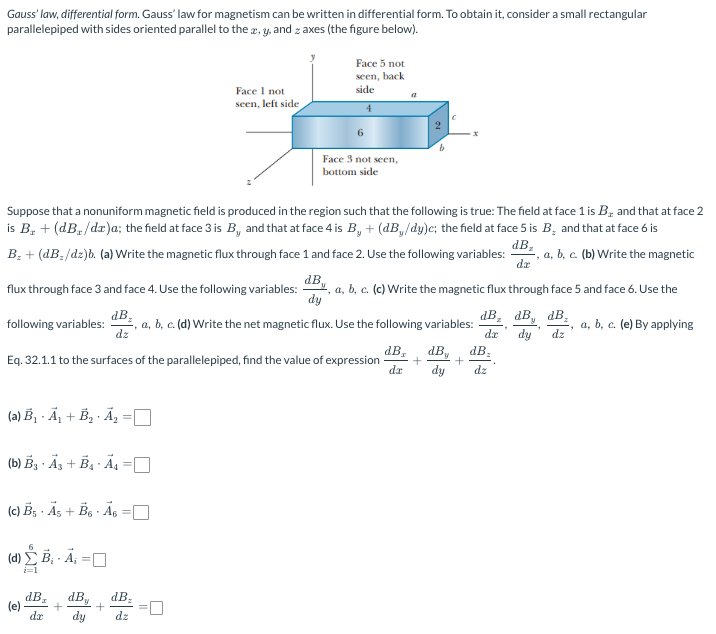
Solved Gauss' law, differential form. Gauss' law for
It states that the divergence of the electric field at any. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. Gauss' law in differential form lends itself most easily to.
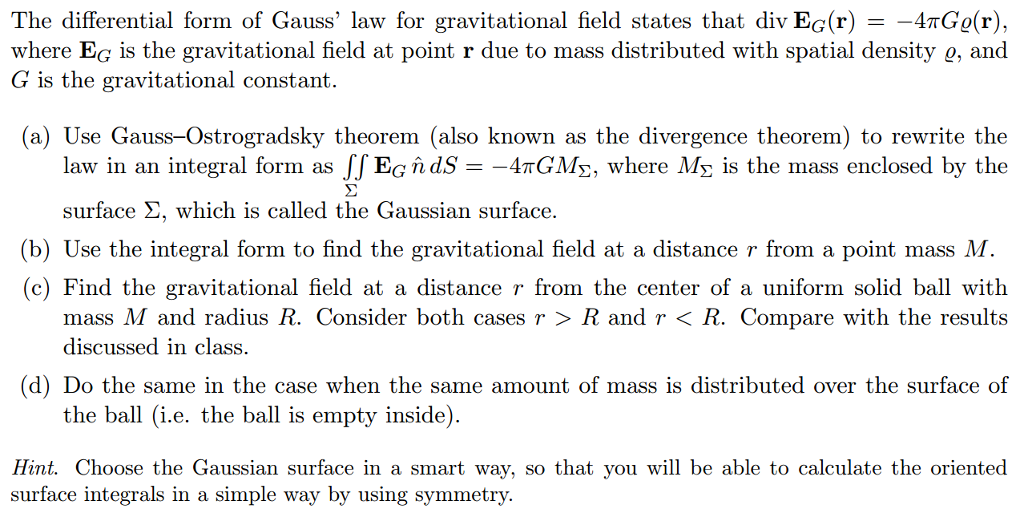
Solved The differential form of Gauss' law for gravitational
This conclusion is the differential form of gauss' law, and is one of maxwell's equations. It states that the divergence of the electric field at any. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. The differential form of gauss law relates the electric field to the charge distribution at.
Solved Gauss's law in differential form relates the electric
(1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. This conclusion is the differential form of gauss' law, and is one of maxwell's equations. Gauss' law in differential form lends.
SOLVED (a) Define Gauss Law of Electrostatics in Differential form. (b
It states that the divergence of the electric field at any. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. The differential form of gauss law relates the electric field.
It States That The Divergence Of The Electric Field At Any.
The differential form of gauss law relates the electric field to the charge distribution at a particular point in space. Gauss' law in differential form lends itself most easily to finding the charge density when we are give the field. (1) in the following part, we will discuss the difference between the integral and differential form of gauss’s law. This conclusion is the differential form of gauss' law, and is one of maxwell's equations.