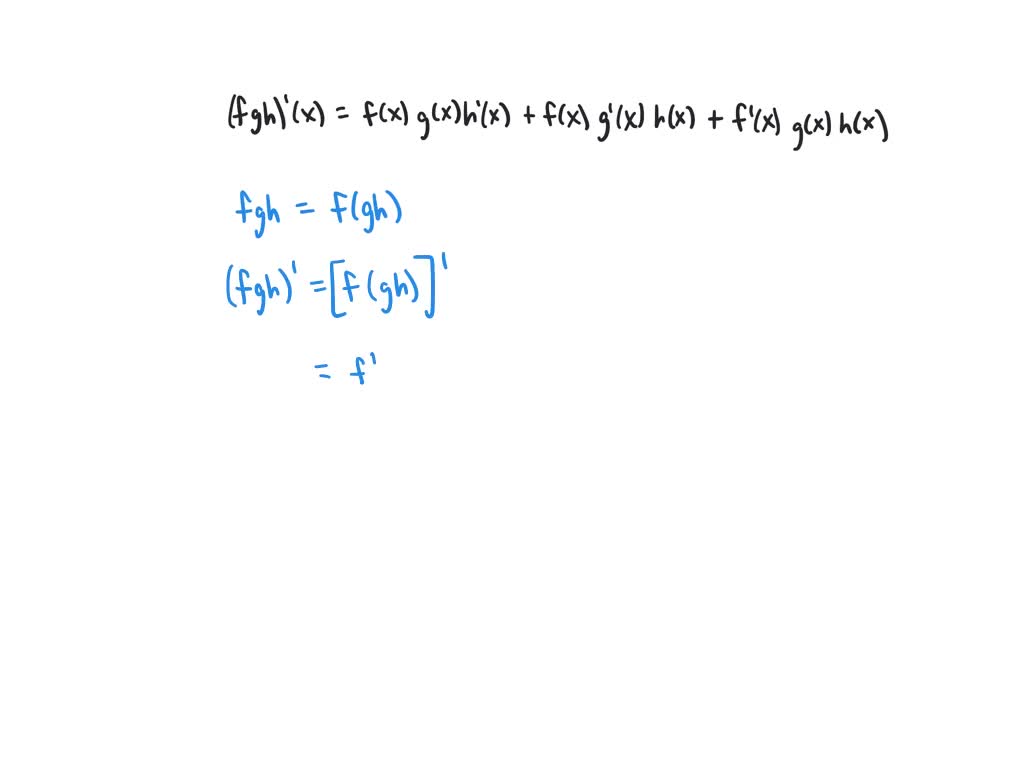Functions F G H And J Are Continuous And Differentiable - If a function is differentiable on an. H (x) = f (x) g (x) and j (x) = g (f (x)). The function has to be continuous. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. The function f, g, h and j is continuous and differentiates for all real numbers. The derivative must exist at each point in the domain of the function.
The function has to be continuous. The function f, g, h and j is continuous and differentiates for all real numbers. The derivative must exist at each point in the domain of the function. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. H (x) = f (x) g (x) and j (x) = g (f (x)). Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. If a function is differentiable on an.
Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. If a function is differentiable on an. The derivative must exist at each point in the domain of the function. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. The function f, g, h and j is continuous and differentiates for all real numbers. The function has to be continuous. H (x) = f (x) g (x) and j (x) = g (f (x)).
Solved Functions f, g, and h continuous and differentiable
If a function is differentiable on an. The function has to be continuous. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. The function f, g, h and.
4.5 continuous functions and differentiable functions
The function has to be continuous. The derivative must exist at each point in the domain of the function. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. H (x) = f (x) g (x) and j (x) = g (f (x)). The function f, g,.
Solved If f, g, and h are differentiable functions, find
The derivative must exist at each point in the domain of the function. H (x) = f (x) g (x) and j (x) = g (f (x)). If a function is differentiable on an. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. The function has.
[Solved] (1) Functions f, g, and h are continuous and differentiable
The function has to be continuous. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. The function f, g, h and j is continuous and differentiates for all real numbers. If a function is differentiable on an. The derivative must exist at each point in the.
4.5 continuous functions and differentiable functions
Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. H (x) = f (x) g (x) and j (x) = g (f (x)). The function f, g, h and j is continuous and differentiates for all real numbers. The derivative must exist at each point in.
SOLVEDLet f, g, h be differentiable functions. Show that (f g h)^'(x
The function f, g, h and j is continuous and differentiates for all real numbers. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. The function has to be continuous. The derivative must exist at each point in the domain of the function. H (x) =.
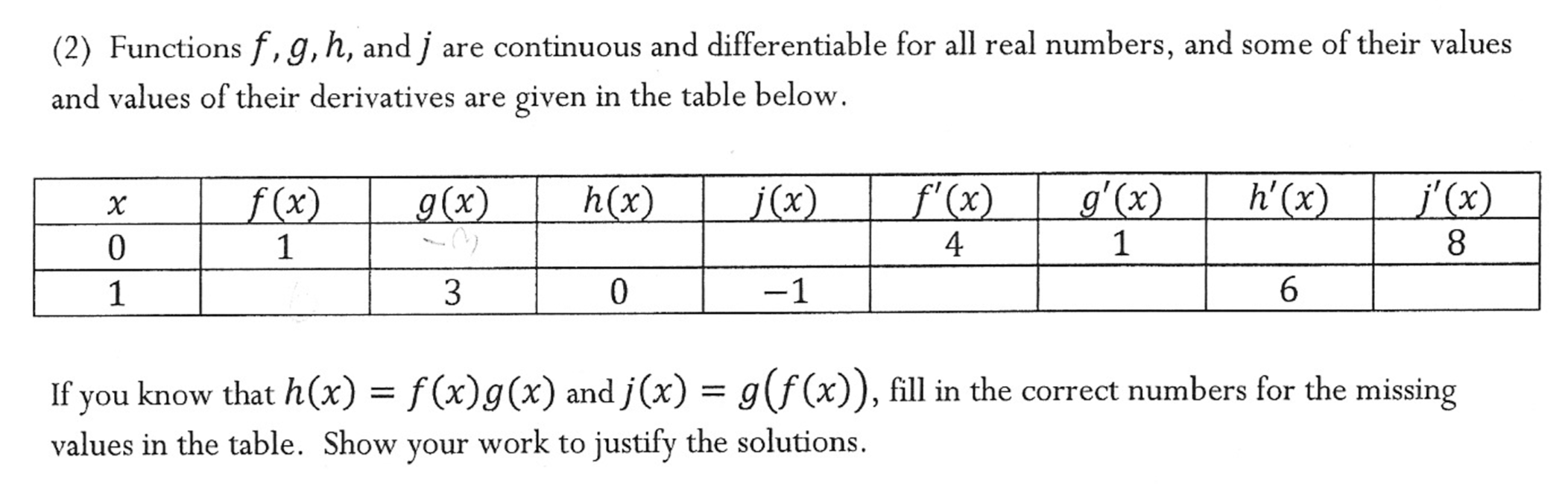
Solved (2) Functions f, g, h, and j are continuous and
If a function is differentiable on an. The function has to be continuous. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. The derivative must exist at each.
Solved (2) Functions f.g,h, and j are continuous and
If a function is differentiable on an. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. The function f, g, h and j is continuous and differentiates for all real numbers. The derivative must exist at each point in the domain of the function. The function has to be continuous.
Solved Functions f,g,h and j are continuous and
The function f, g, h and j is continuous and differentiates for all real numbers. H (x) = f (x) g (x) and j (x) = g (f (x)). The function has to be continuous. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. The derivative must exist at each point.
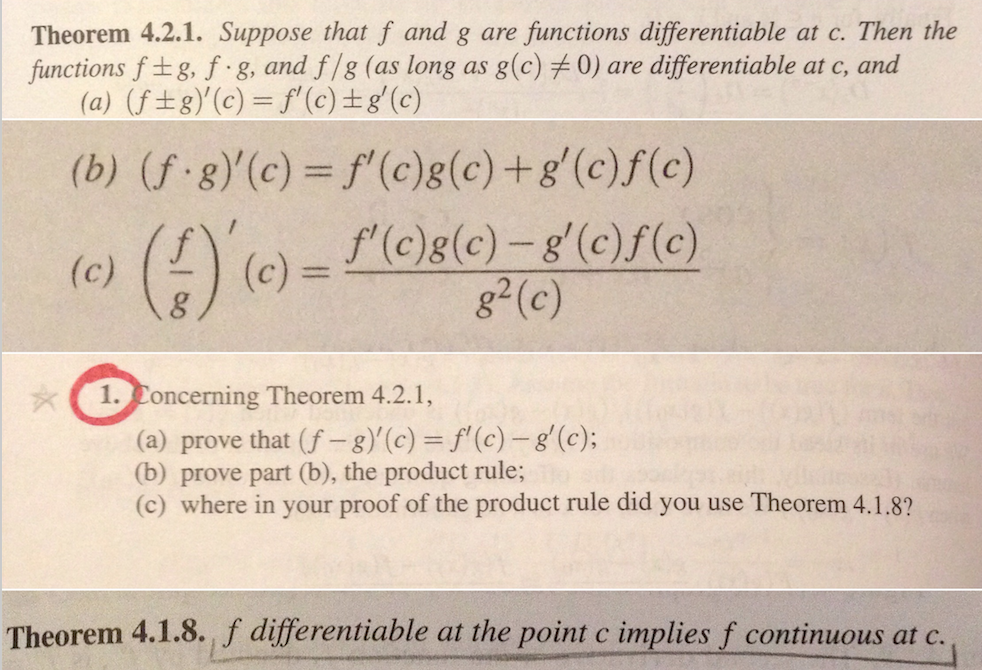
Solved Suppose that f and g are functions differentiable at
The function has to be continuous. The function f, g, h and j is continuous and differentiates for all real numbers. The derivative must exist at each point in the domain of the function. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. Functions f,g,h and j are continuous and differentiable.
If A Function Is Differentiable On An.
The derivative must exist at each point in the domain of the function. Show that if $x_0$ is in $j$, $h:j\rightarrow\mathbb{r}$ is continuous at $x_0$, $h(x)\neq h(x_0)$ if $x\neq x_0$, and. Functions f,g,h and j are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are. H (x) = f (x) g (x) and j (x) = g (f (x)).
The Function F, G, H And J Is Continuous And Differentiates For All Real Numbers.
The function has to be continuous.