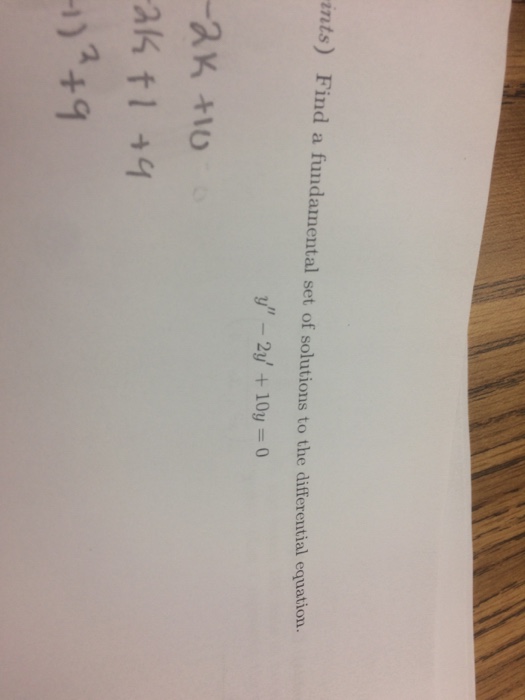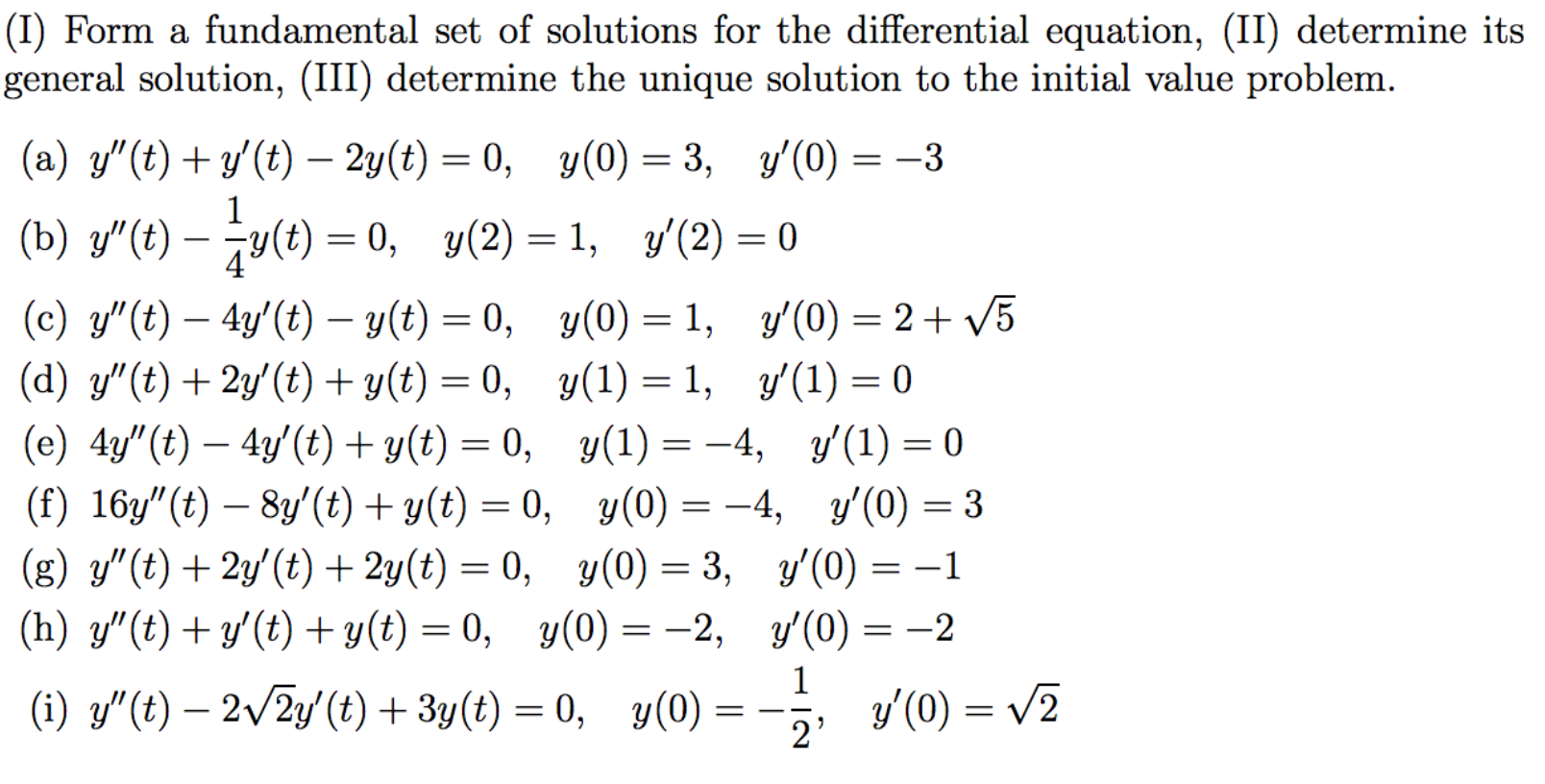Find The Fundamental Set Of Solutions For The Differential Equation - Now substitute each of these functions into the. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. Put in another way, every. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second.
Put in another way, every. Now substitute each of these functions into the. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t.
• find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. Now substitute each of these functions into the. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. Put in another way, every. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t.
[Solved] Find the fundamental set of solutions for the differential
(a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. A fundamental set of solutions to a differential equation is the.
(PDF) PROBLEM SET & SOLUTIONS DIFFERENTIAL EQUATION
(a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\..
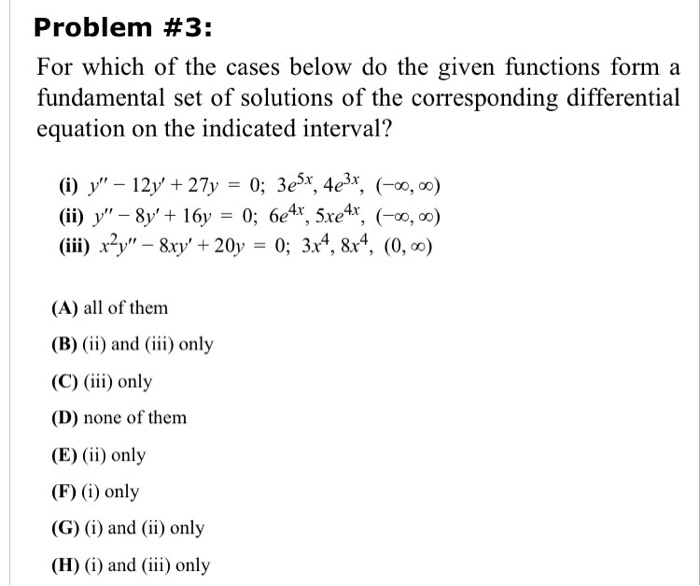
Solved For which of the cases below do the given functions
The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. Put in another way, every. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. We.
Solved Find the fundamental set of solutions to the
A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. Now substitute each of these functions into the. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. • find the fundamental set specified by theorem 3.2.5.
Solved Form a fundamental set of solutions for the
A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. Now substitute each of these functions into the. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we.
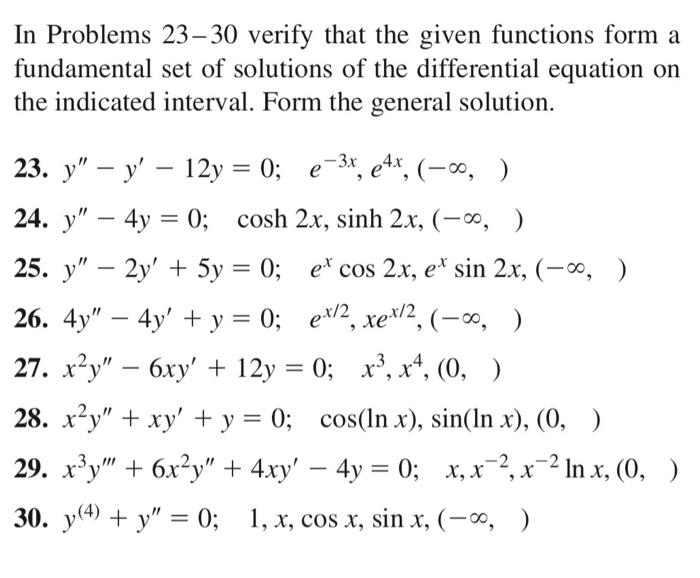
Solved In Problems 2330 verify that the given functions
(a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. Now substitute each of these functions into the. Put in another way, every. • find the fundamental set specified by theorem 3.2.5 for the differential equation.
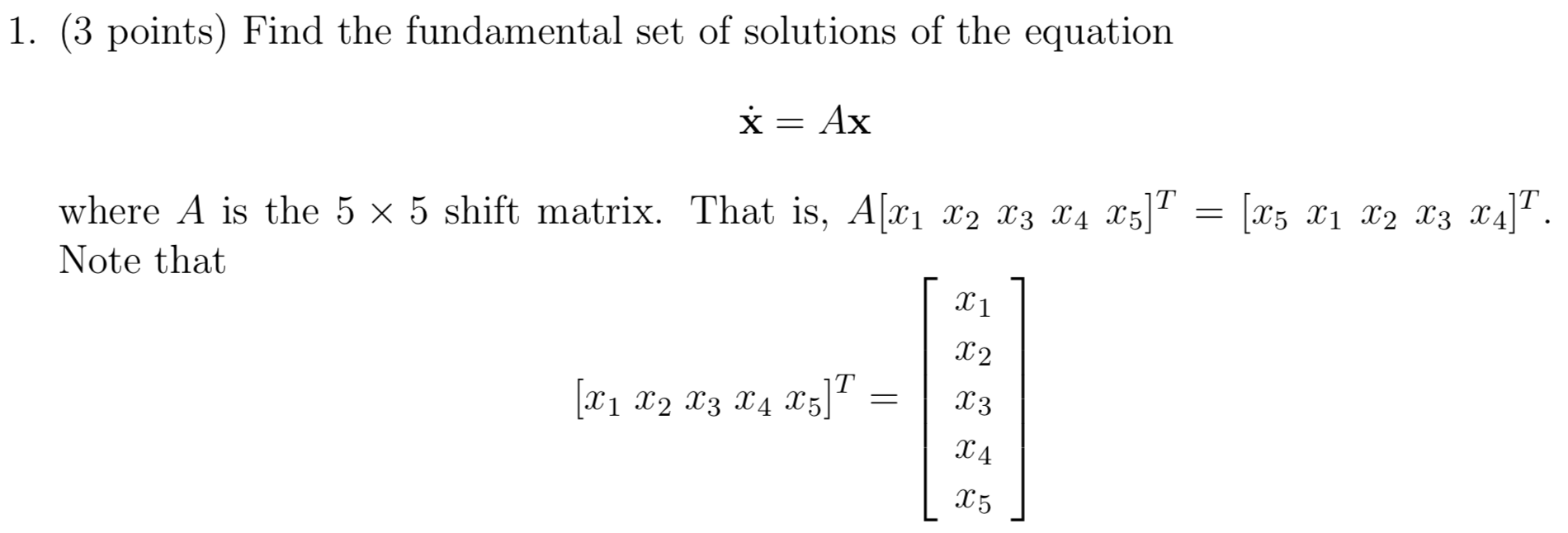
Solved 1. (3 points) Find the fundamental set of solutions
A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. (a) no (b) to be a general.
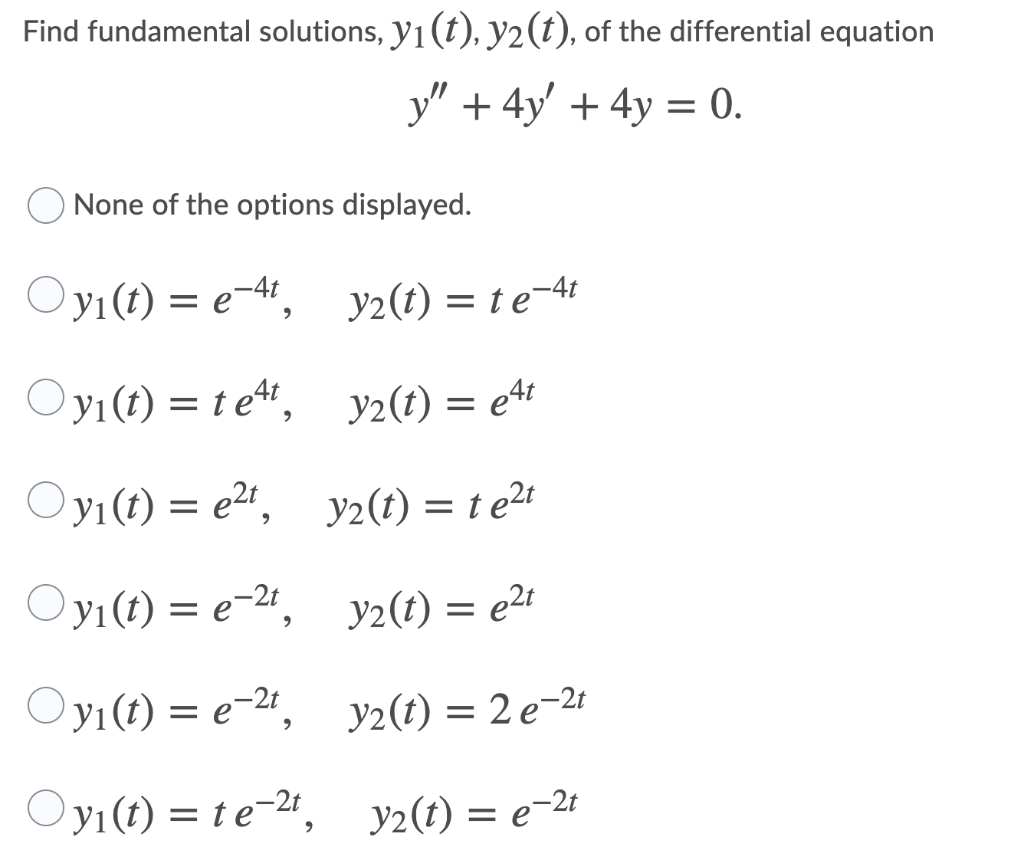
Find fundamental solutions, yı(t), y2(t), of the
The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. We define fundamental sets of solutions and discuss how they can be used to get a general solution to a homogeneous second. Now substitute each of these functions into.
Solved Find realvalued fundamental solutions of the
Put in another way, every. (a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. •.
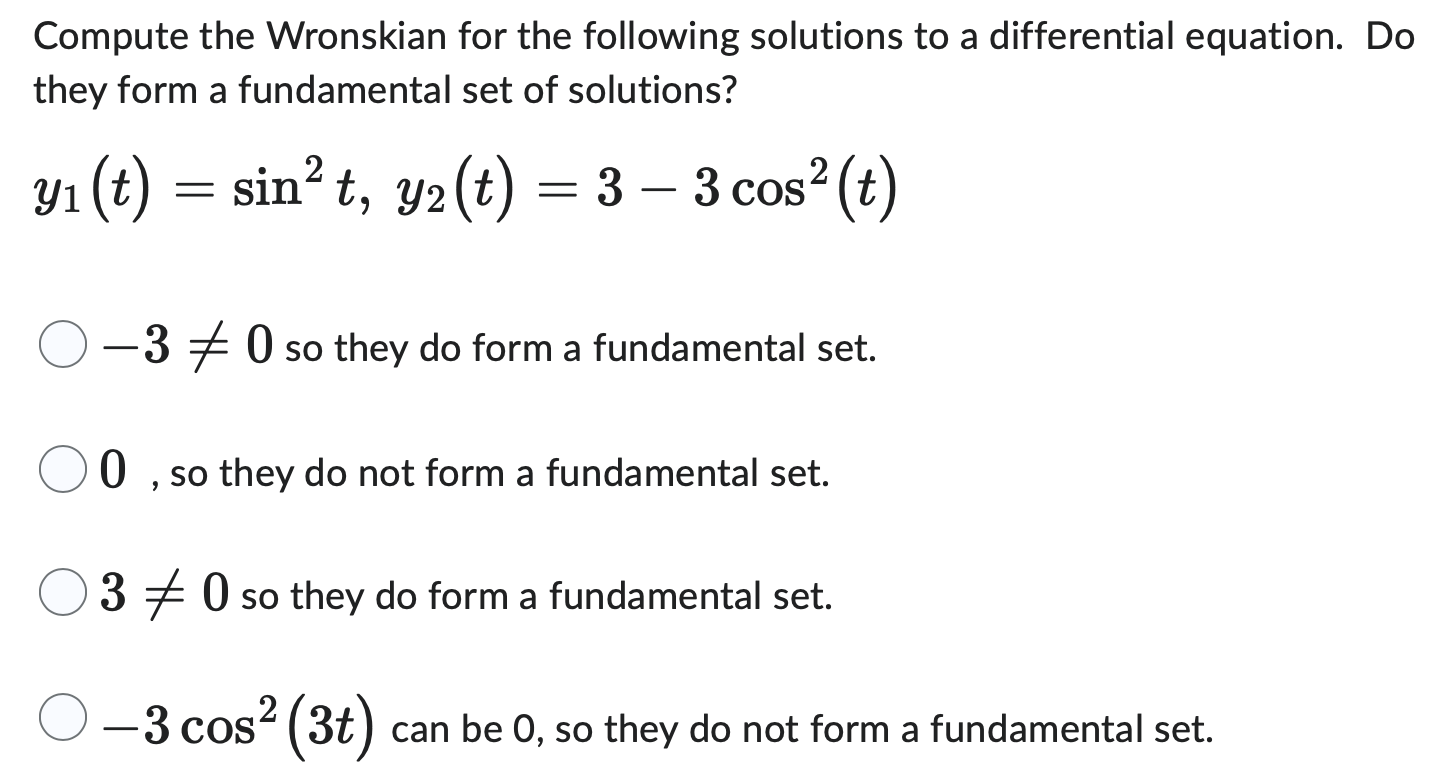
Solved Compute the Wronskian for the following solutions to
A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. (a) no (b).
We Define Fundamental Sets Of Solutions And Discuss How They Can Be Used To Get A General Solution To A Homogeneous Second.
Now substitute each of these functions into the. The general solution of this system of differential equations is $$ae^{x}v_1+be^{2x}v_2=\begin{pmatrix}ae^x+be^{2x}\\. A fundamental set of solutions to a differential equation is the basis of the solution space of the differential equation. • find the fundamental set specified by theorem 3.2.5 for the differential equation and initial point • in section 3.1, we found two solutions of this.
Put In Another Way, Every.
(a) no (b) to be a general solution, a fundamental set for the equation is s = t cos t, t sin t.