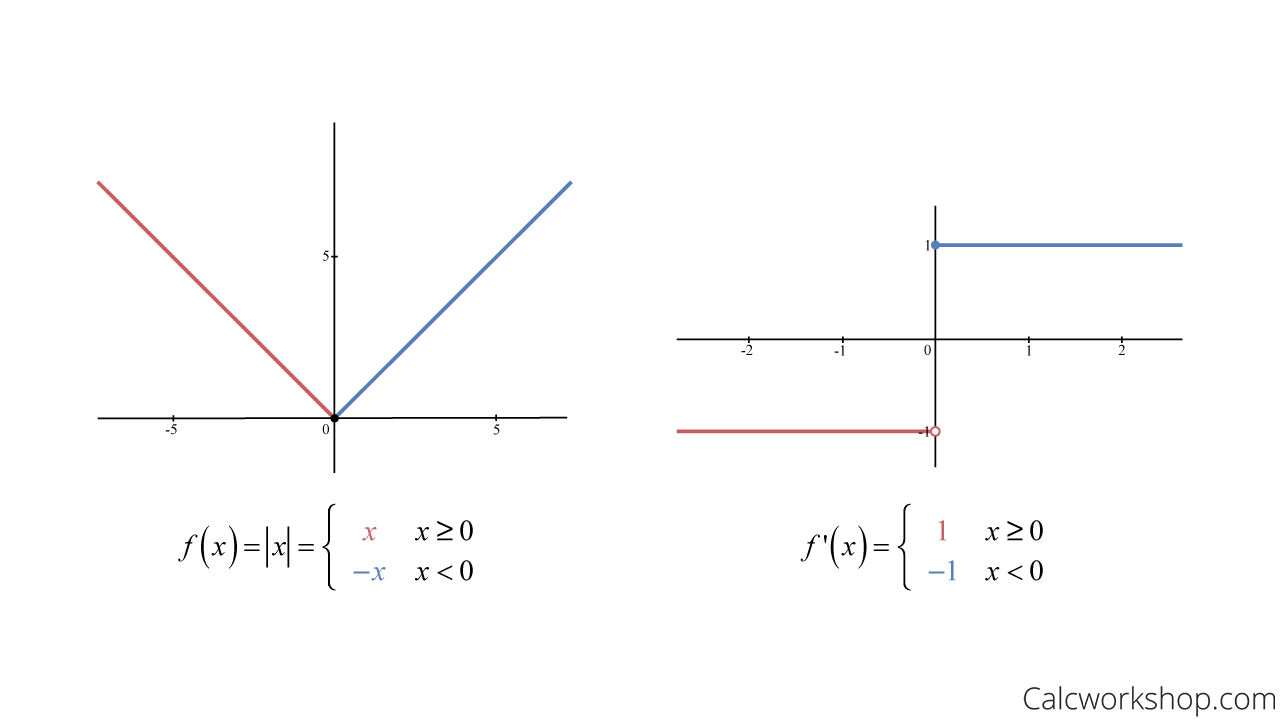
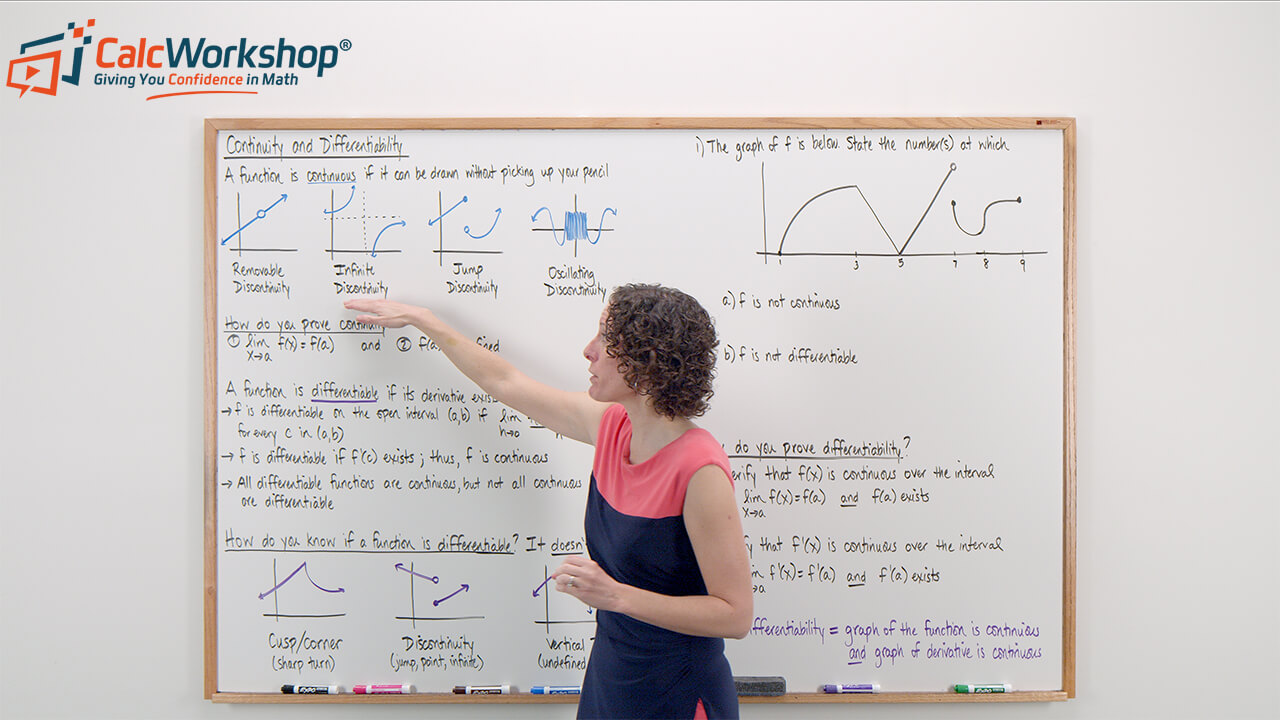
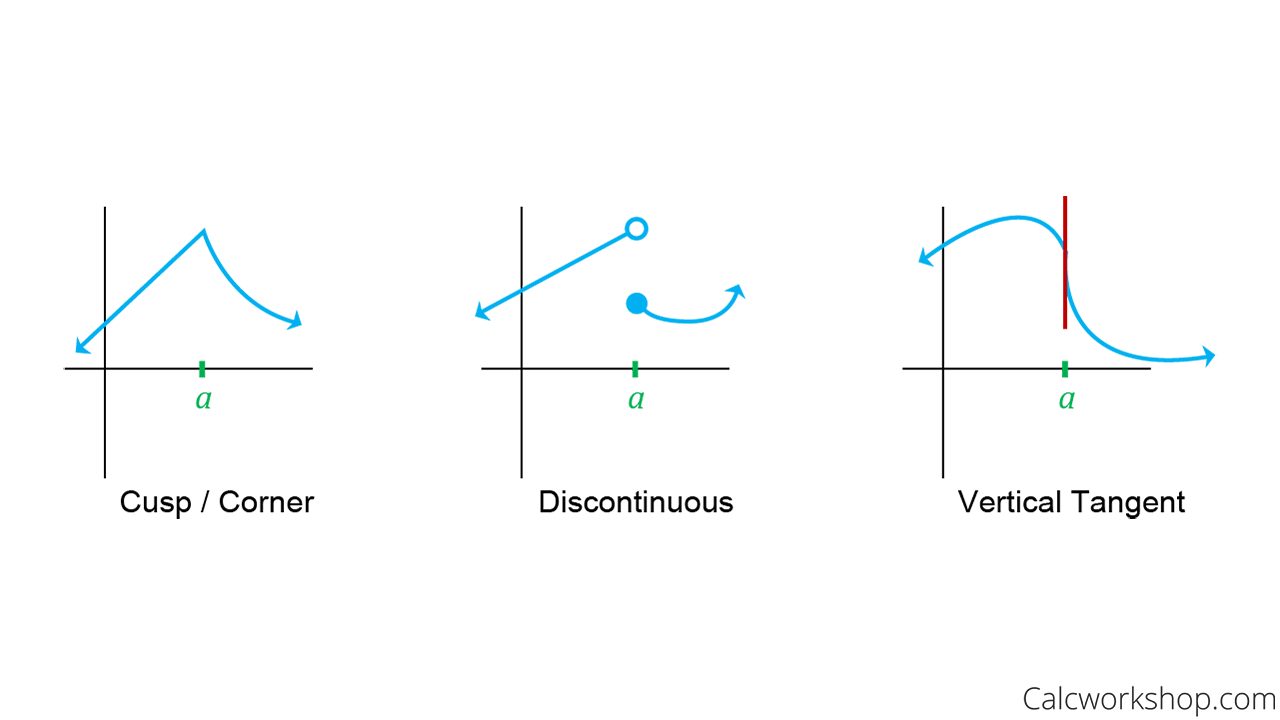
F Is Not Differentiable - The function jumps at x x, (is not continuous) like. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist.
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. The function jumps at x x, (is not continuous) like. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4).
We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. The function jumps at x x, (is not continuous) like. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4).
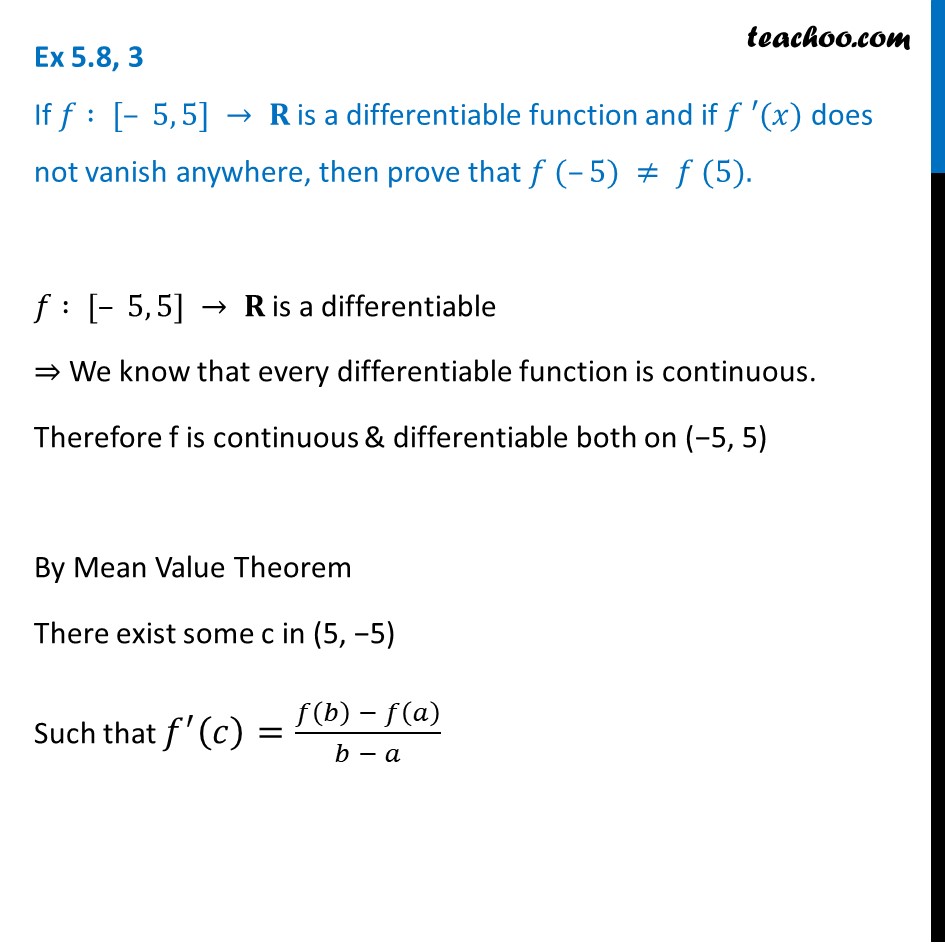
Question 3 If f(x) is differentiable and f'(x) does not vanish
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. A function f.
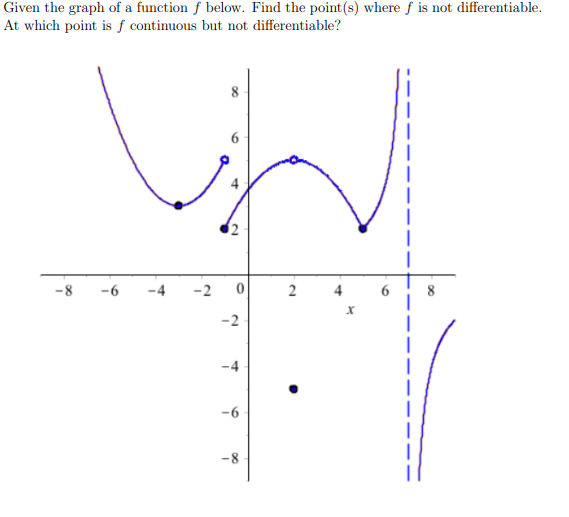
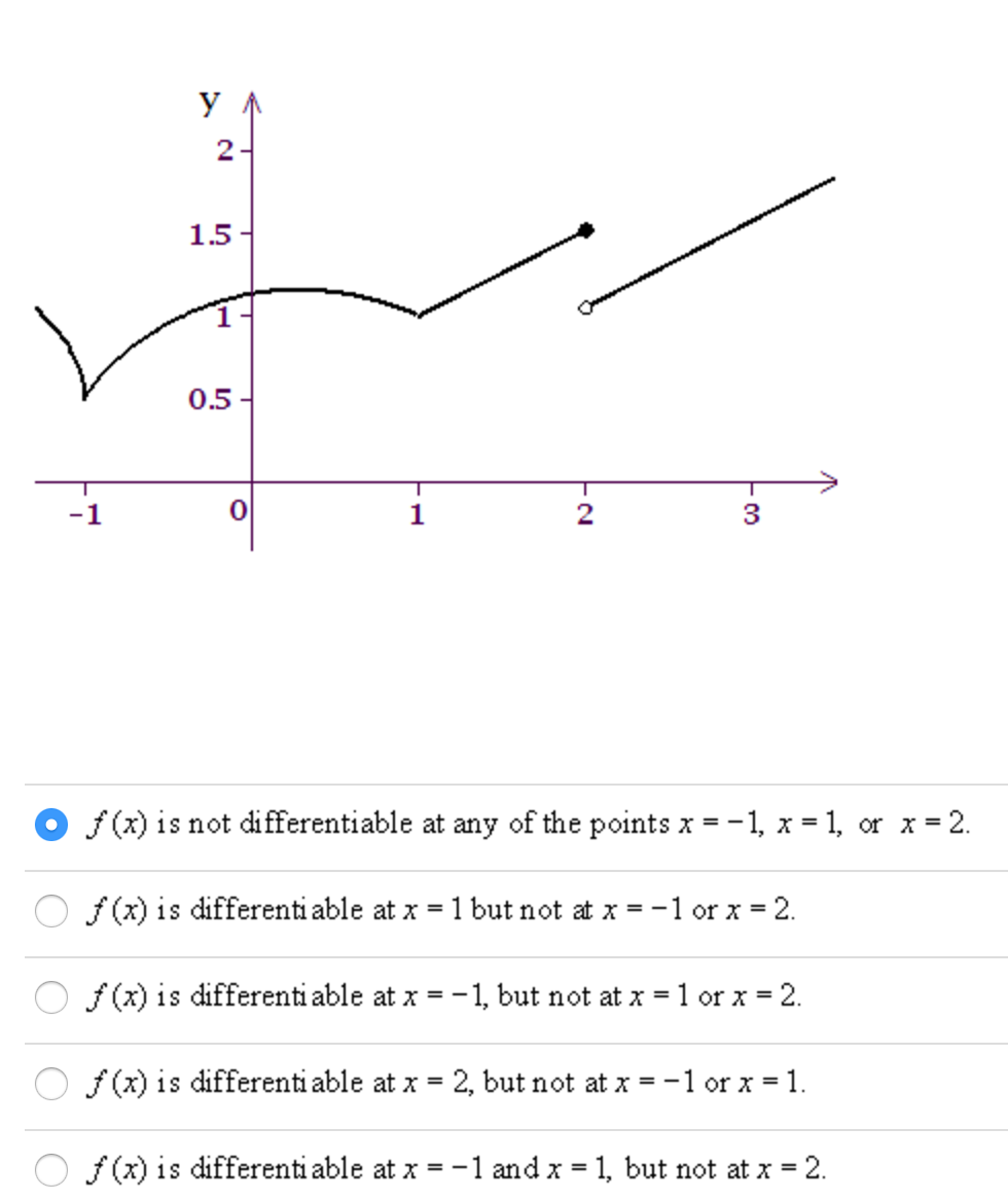
given the graph of a function f below find the points where f is not
A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but.
Where Is F Not Differentiable
We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. The function jumps at x x, (is not continuous) like. A function f.
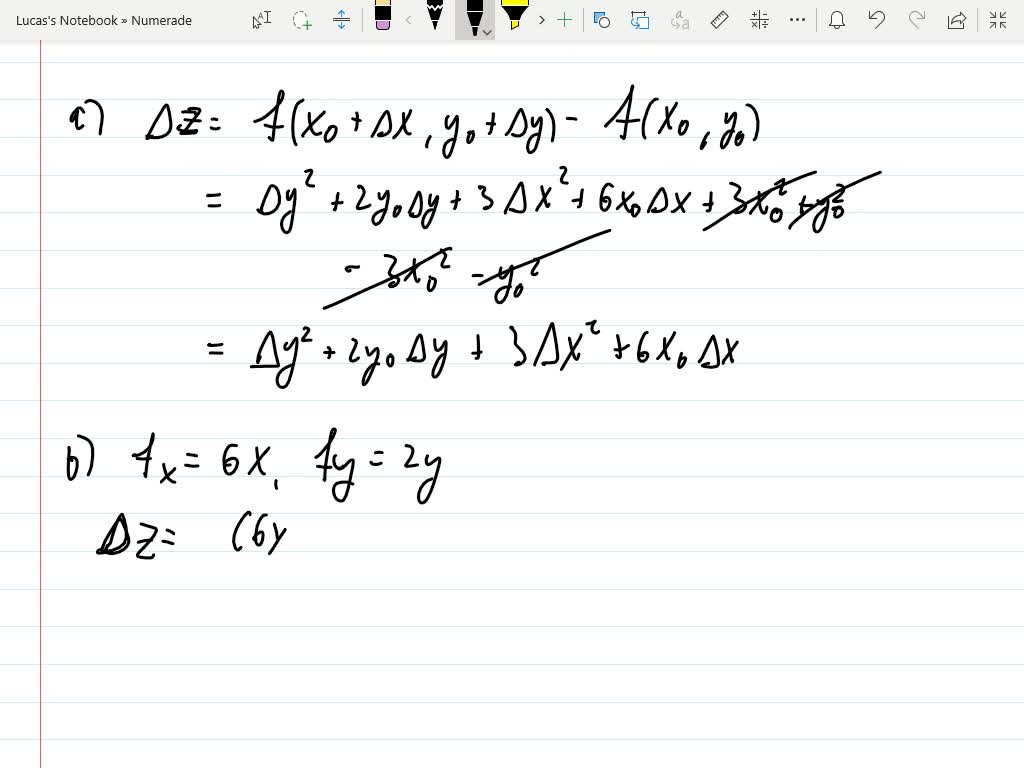
A function f(z) is given. For each, (a) where is f differentiable? (b
A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. The function jumps at x x, (is not continuous) like. We can say that.
calculus Show f is not differentiable at x=0 Mathematics Stack Exchange
The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph.
Is F(X) = X Differentiable? Exploring The Derivative Of A Simple Function
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. The function jumps at x x, (is not continuous) like. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). We can say that.
If F X is Differentiable Then F X is Continuous Flowers Hishe1974
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). The function jumps at x x, (is not continuous) like. We can say that.
Question 3 If f(x) is differentiable and f'(x) does not vanish
The function jumps at x x, (is not continuous) like. But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. A function f.
Solved f(x) is not differentiable at any of the points x =
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. A function f is not differentiable if (1) f is not continuous (2).
If F X is Differentiable Then F X is Continuous Flowers Hishe1974
The function jumps at x x, (is not continuous) like. But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4). We can say that.
The Function Jumps At X X, (Is Not Continuous) Like.
But the function \(f\) in figure \(\pageindex{6}\) is not differentiable at \(a = 1\) because \(f'(1)\) fails to exist. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. A function f is not differentiable if (1) f is not continuous (2) left and right derivatives are different (3) the graph has a vertical tangent (4).