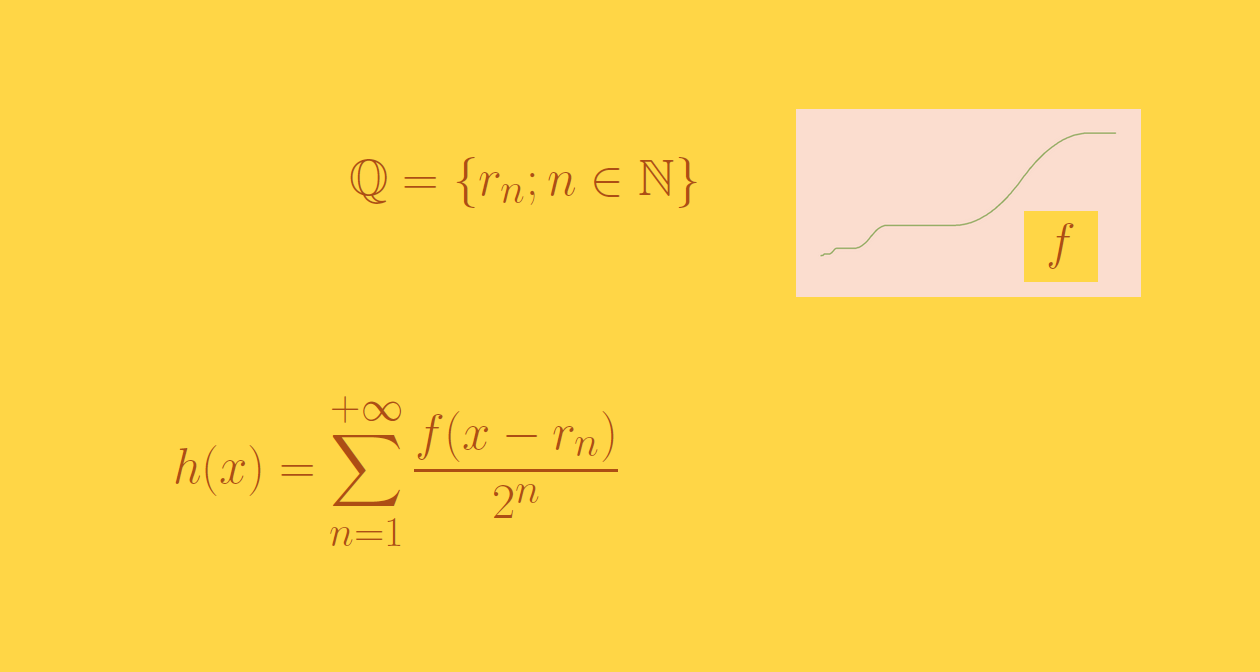Every Continuous Function Is Differentiable True Or False - The correct option is b false. Every continuous function is always differentiable. Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. [a, b] → r f: So it may seem reasonable that all continuous functions are differentiable a.e. This turns out to be. If a function f (x) is differentiable at a point a, then it is continuous at the point a. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). [a, b] → r be continuously differentiable. Let us take an example function which will result into the testing of statement.
[a, b] → r be continuously differentiable. This turns out to be. [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$. [a, b] → r f: The correct option is b false. If a function f (x) is differentiable at a point a, then it is continuous at the point a. But we see that $f(x) = |x|$ is continuous because $\mathop {\lim }\limits_{x \to c} f(x) = \mathop {\lim }\limits_{x \to c} |x| = f(c)$ exists for all. So it may seem reasonable that all continuous functions are differentiable a.e. Every continuous function is always differentiable. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable).
But we see that $f(x) = |x|$ is continuous because $\mathop {\lim }\limits_{x \to c} f(x) = \mathop {\lim }\limits_{x \to c} |x| = f(c)$ exists for all. Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. So it may seem reasonable that all continuous functions are differentiable a.e. If a function f (x) is differentiable at a point a, then it is continuous at the point a. Let us take an example function which will result into the testing of statement. This turns out to be. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). [a, b] → r be continuously differentiable. [a, b] → r f: [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$.
acontinuousfunctionnotdifferentiableontherationals
[a, b] → r be continuously differentiable. [a, b] → r f: So it may seem reasonable that all continuous functions are differentiable a.e. [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$. But we see that $f(x) = |x|$ is continuous because $\mathop {\lim }\limits_{x \to c} f(x) = \mathop {\lim }\limits_{x \to.
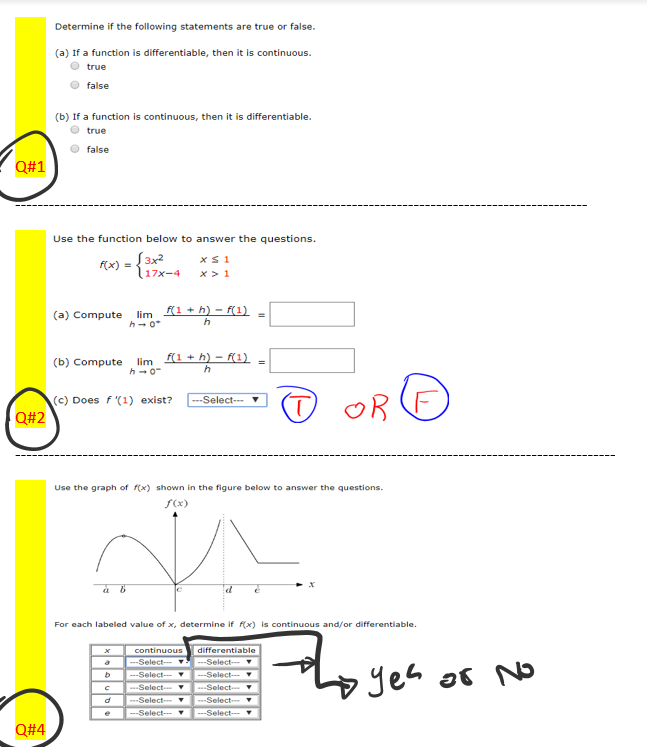
Solved Determine if the following statements are true or
Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. Let us take an example function which will result into the testing of statement. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). This turns out to be. If a function f (x) is.
Solved 1. True Or False If A Function Is Continuous At A...
Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). But we see that $f(x) = |x|$ is continuous because $\mathop {\lim }\limits_{x \to c} f(x) = \mathop {\lim }\limits_{x \to c} |x| = f(c)$.
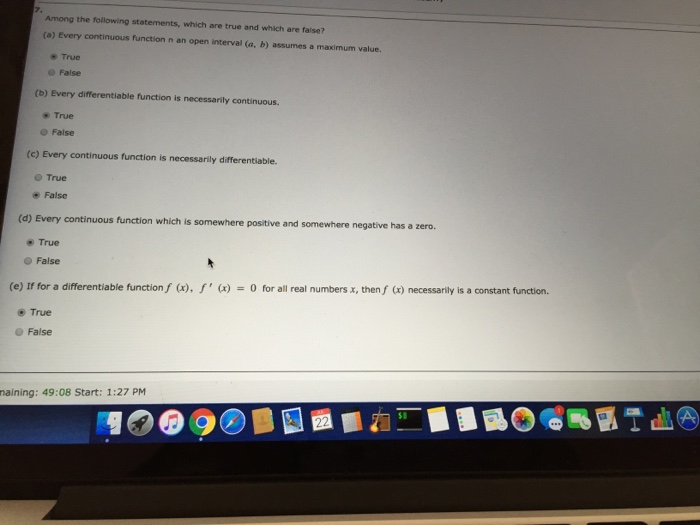
Solved Among the following statements, which are true and
So it may seem reasonable that all continuous functions are differentiable a.e. Let us take an example function which will result into the testing of statement. [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$. [a, b] → r f: Show that every differentiable function is continuous (converse is not true i.e., a function.
Differentiable vs. Continuous Functions Understanding the Distinctions
But we see that $f(x) = |x|$ is continuous because $\mathop {\lim }\limits_{x \to c} f(x) = \mathop {\lim }\limits_{x \to c} |x| = f(c)$ exists for all. If a function f (x) is differentiable at a point a, then it is continuous at the point a. The correct option is b false. [a, b] → r f: Then its.
Solved III. 25. Which of the following is/are true? 1. Every
This turns out to be. If a function f (x) is differentiable at a point a, then it is continuous at the point a. The correct option is b false. [a, b] → r f: Every continuous function is always differentiable.
VIDEO solution If a function of f is differentiable at x=a then the
Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). So it may seem reasonable that all continuous functions are differentiable a.e. Every continuous function is always differentiable. [a, b] → r be continuously differentiable. [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$.
Solved If f is continuous at a number, x, then f is differentiable at
Let us take an example function which will result into the testing of statement. [a, b] → r f: So it may seem reasonable that all continuous functions are differentiable a.e. This turns out to be. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable).
Solved QUESTION 32 Every continuous function is
[a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$. Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. [a, b] → r be continuously differentiable. This turns out to be. Every continuous function is always differentiable.
SOLVED 'True or False If a function is continuous at point; then it
The correct option is b false. Then its derivative f′ f ′ is continuous on [a, b] [a, b] and hence. Let us take an example function which will result into the testing of statement. [a, b] → r be continuously differentiable. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but.
Then Its Derivative F′ F ′ Is Continuous On [A, B] [A, B] And Hence.
[a, b] → r be continuously differentiable. [a,b]\to {\mathbb r}$ which equals $0$ at $a$, and equals $1$ on the interval $(a,b]$. Every continuous function is always differentiable. This turns out to be.
But We See That $F(X) = |X|$ Is Continuous Because $\Mathop {\Lim }\Limits_{X \To C} F(X) = \Mathop {\Lim }\Limits_{X \To C} |X| = F(C)$ Exists For All.
The correct option is b false. So it may seem reasonable that all continuous functions are differentiable a.e. Show that every differentiable function is continuous (converse is not true i.e., a function may be continuous but not differentiable). If a function f (x) is differentiable at a point a, then it is continuous at the point a.
[A, B] → R F:
Let us take an example function which will result into the testing of statement.