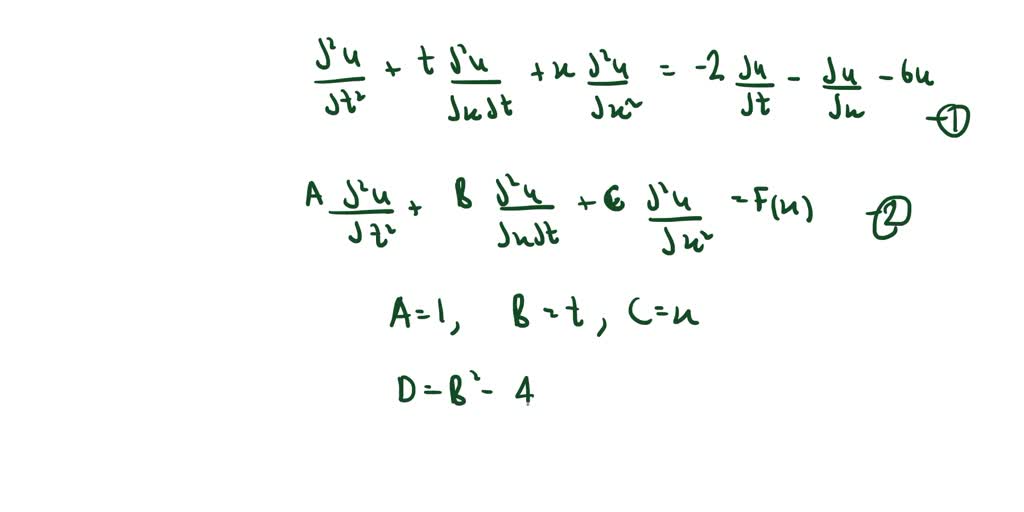Elliptic Differential Equation - This could model the temperature distribution on a square floor. Primarily the dirichlet problem for various types of elliptic equations. Lu= xn i,j=1 a ij(x)∂ iju(a non. Elliptic partial differential equations by qing. Thus, the laplace equation is elliptic. Praise for the first edition: Differential operator of one of the two forms: Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. A solution to this equation is u(x;
Lu= xn i,j=1 a ij(x)∂ iju(a non. Praise for the first edition: Thus, the laplace equation is elliptic. Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Primarily the dirichlet problem for various types of elliptic equations. Differential operator of one of the two forms: A solution to this equation is u(x; This could model the temperature distribution on a square floor. Elliptic partial differential equations by qing.
Primarily the dirichlet problem for various types of elliptic equations. Lu= xn i,j=1 a ij(x)∂ iju(a non. Thus, the laplace equation is elliptic. A solution to this equation is u(x; Praise for the first edition: Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. This could model the temperature distribution on a square floor. Differential operator of one of the two forms: Elliptic partial differential equations by qing.
Lecture 7_elliptic partial differential equation PDF Equations
Thus, the laplace equation is elliptic. Differential operator of one of the two forms: Primarily the dirichlet problem for various types of elliptic equations. Elliptic partial differential equations by qing. Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2.
MAFE208IUL14 Elliptic Equation PDF Partial Differential Equation
Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Lu= xn i,j=1 a ij(x)∂ iju(a non. Elliptic partial differential equations by qing. This could model the temperature distribution on a square floor. Praise for the first edition:
Online video course design of elliptic partial differential equation
This could model the temperature distribution on a square floor. Lu= xn i,j=1 a ij(x)∂ iju(a non. Primarily the dirichlet problem for various types of elliptic equations. Praise for the first edition: A solution to this equation is u(x;
Solved Solve the elliptic partial differential equation
Lu= xn i,j=1 a ij(x)∂ iju(a non. Praise for the first edition: Elliptic partial differential equations by qing. Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Primarily the dirichlet problem for various types of elliptic equations.
(PDF) Analytic regularity and solution approximation for a semilinear
Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Lu= xn i,j=1 a ij(x)∂ iju(a non. Thus, the laplace equation is elliptic. Praise for the first edition: A solution to this equation is u(x;
Solved 5. Which type of differential equation is used to
Praise for the first edition: Differential operator of one of the two forms: This could model the temperature distribution on a square floor. Elliptic partial differential equations by qing. Primarily the dirichlet problem for various types of elliptic equations.
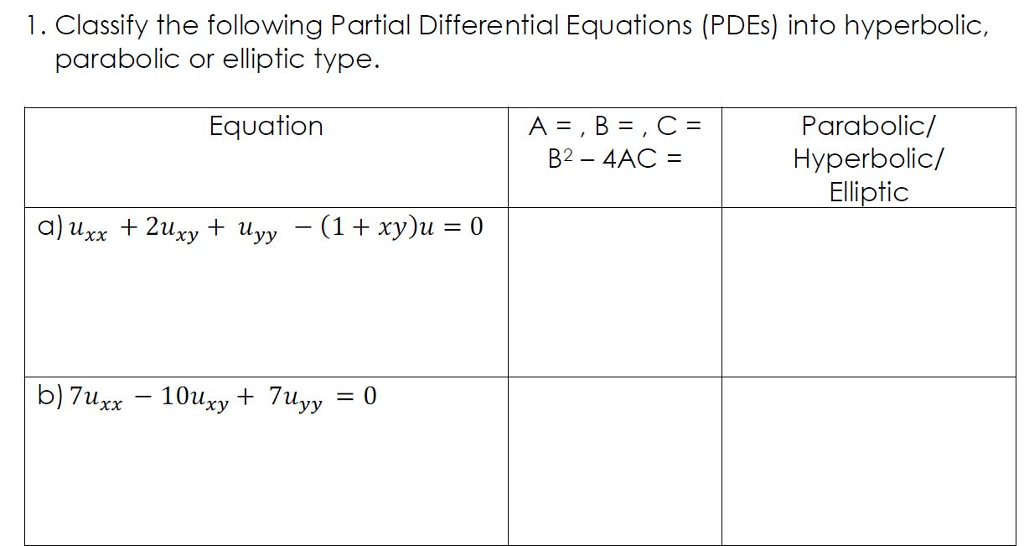
SOLVED Classify the partial differential equation ∂^2u/∂x^2 + 5âˆ
Differential operator of one of the two forms: This could model the temperature distribution on a square floor. Thus, the laplace equation is elliptic. Praise for the first edition: A solution to this equation is u(x;
Boundary value problems for a secondorder elliptic partial
Primarily the dirichlet problem for various types of elliptic equations. Lu= xn i,j=1 a ij(x)∂ iju(a non. Praise for the first edition: A solution to this equation is u(x; Elliptic partial differential equations by qing.
Finalchapter4part4 Pde Elliptic Download Free PDF Partial
Praise for the first edition: This could model the temperature distribution on a square floor. Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Elliptic partial differential equations by qing. Differential operator of one of the two forms:
Solved 1. Classify the following Partial Differential
A solution to this equation is u(x; Lu= xn i,j=1 a ij(x)∂ iju(a non. Praise for the first edition: This could model the temperature distribution on a square floor. Elliptic partial differential equations by qing.
A Solution To This Equation Is U(X;
Praise for the first edition: Lu= xn i,j=1 a ij(x)∂ iju(a non. Lu= xn i,j=1 ∂ i(a ij(x)∂ ju) (a divergence form operator) 2. Elliptic partial differential equations by qing.
Thus, The Laplace Equation Is Elliptic.
Primarily the dirichlet problem for various types of elliptic equations. Differential operator of one of the two forms: This could model the temperature distribution on a square floor.