Dot Product Differentiation - The proof can be extended to any kind of dot product defined. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The dot product of $\mathbf f$ with its derivative is given by: You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +.
$\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The proof can be extended to any kind of dot product defined. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The dot product of $\mathbf f$ with its derivative is given by:
$\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The proof can be extended to any kind of dot product defined. The dot product of $\mathbf f$ with its derivative is given by:
Product Differentiation Glossary ProdPad
You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The dot product of $\mathbf f$ with its derivative is given by: The proof can be extended to any kind of dot product defined.
Product differentiation PPT
The proof can be extended to any kind of dot product defined. The dot product of $\mathbf f$ with its derivative is given by: You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =.
Product differentiation PPT
The proof can be extended to any kind of dot product defined. The dot product of $\mathbf f$ with its derivative is given by: You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =.
Product differentiation PPT
$\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The dot product of $\mathbf f$ with its derivative is given by: The proof can be extended to any kind of dot product defined. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +.
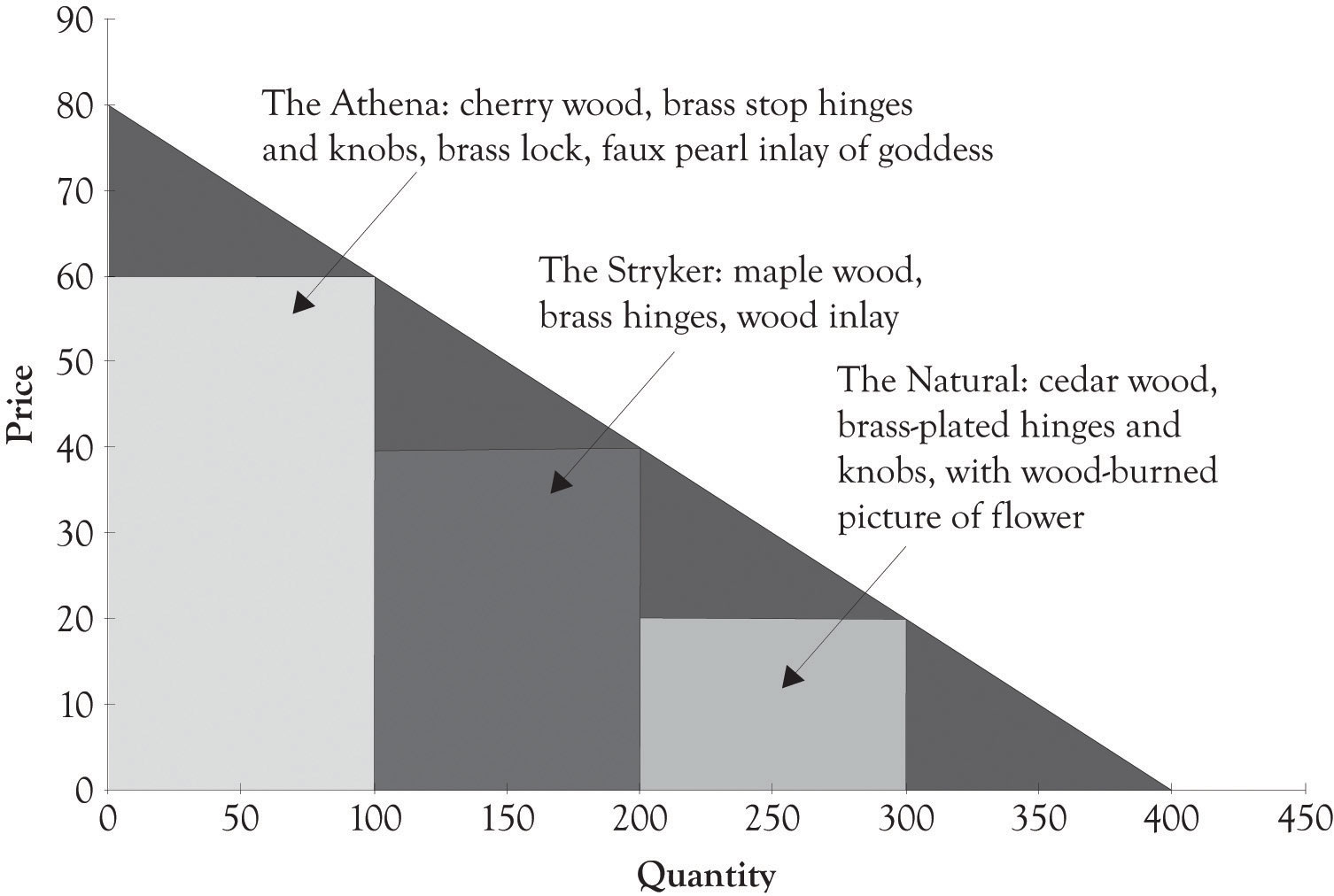
Product Differentiation Curves
The dot product of $\mathbf f$ with its derivative is given by: The proof can be extended to any kind of dot product defined. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =.
Dot Product dan cross product PDF
$\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The dot product of $\mathbf f$ with its derivative is given by: The proof can be extended to any kind of dot product defined.
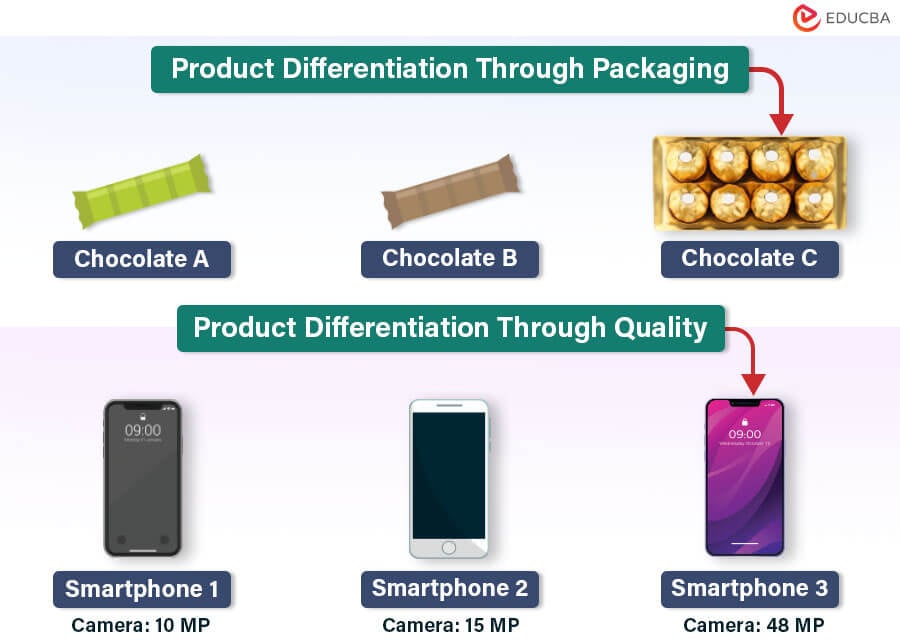
Fresh Approach to Product Differentiation
You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The dot product of $\mathbf f$ with its derivative is given by: $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The proof can be extended to any kind of dot product defined.
Product Differentiation in Marketing Definition, Real Examples
The dot product of $\mathbf f$ with its derivative is given by: You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. The proof can be extended to any kind of dot product defined.
The Dot Product Definition and Example Math lab, Algebra, Mathematics
The dot product of $\mathbf f$ with its derivative is given by: $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The proof can be extended to any kind of dot product defined.
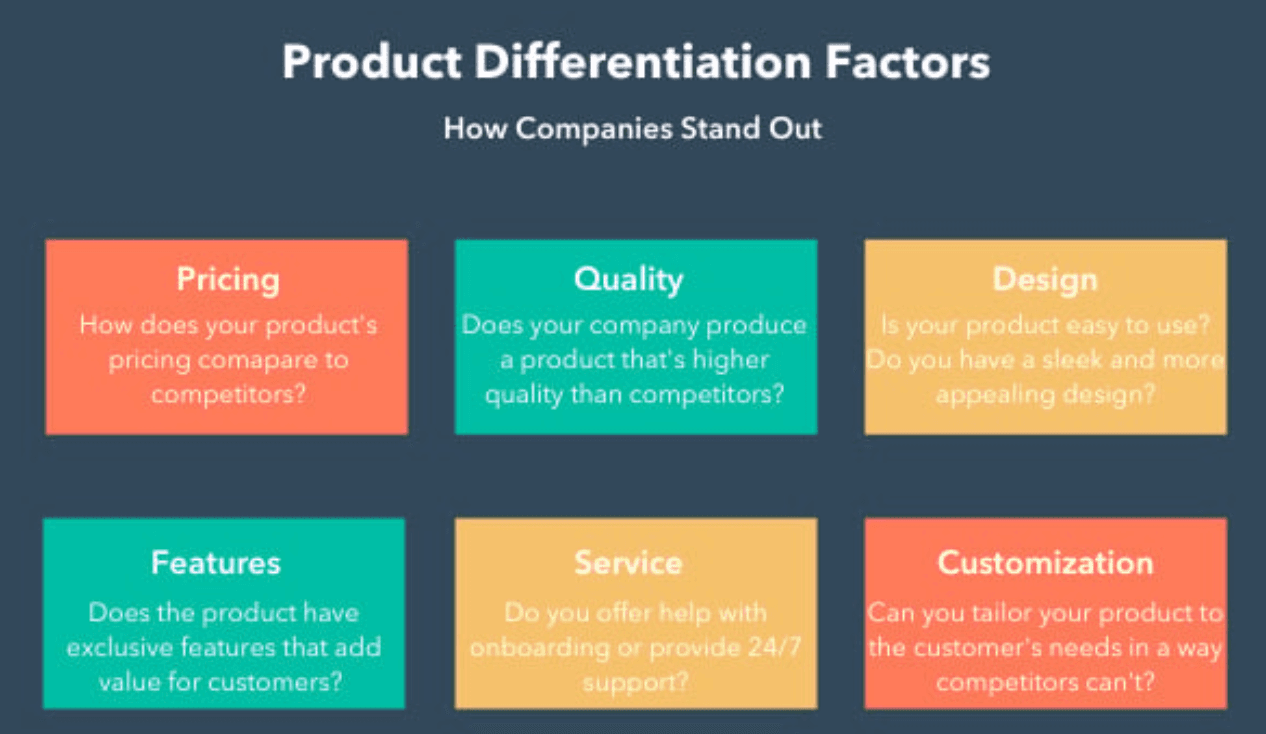
What Is Product Differentiation? Ultimate Marketing Dictionary
The proof can be extended to any kind of dot product defined. $\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The dot product of $\mathbf f$ with its derivative is given by:
The Proof Can Be Extended To Any Kind Of Dot Product Defined.
$\map {\mathbf f} x \cdot \dfrac {\map {\d \mathbf f} x} {\d x} =. You're assuming the dot product is x ∗ y = x0y0 + x1y1 + x2y2 +. The dot product of $\mathbf f$ with its derivative is given by: