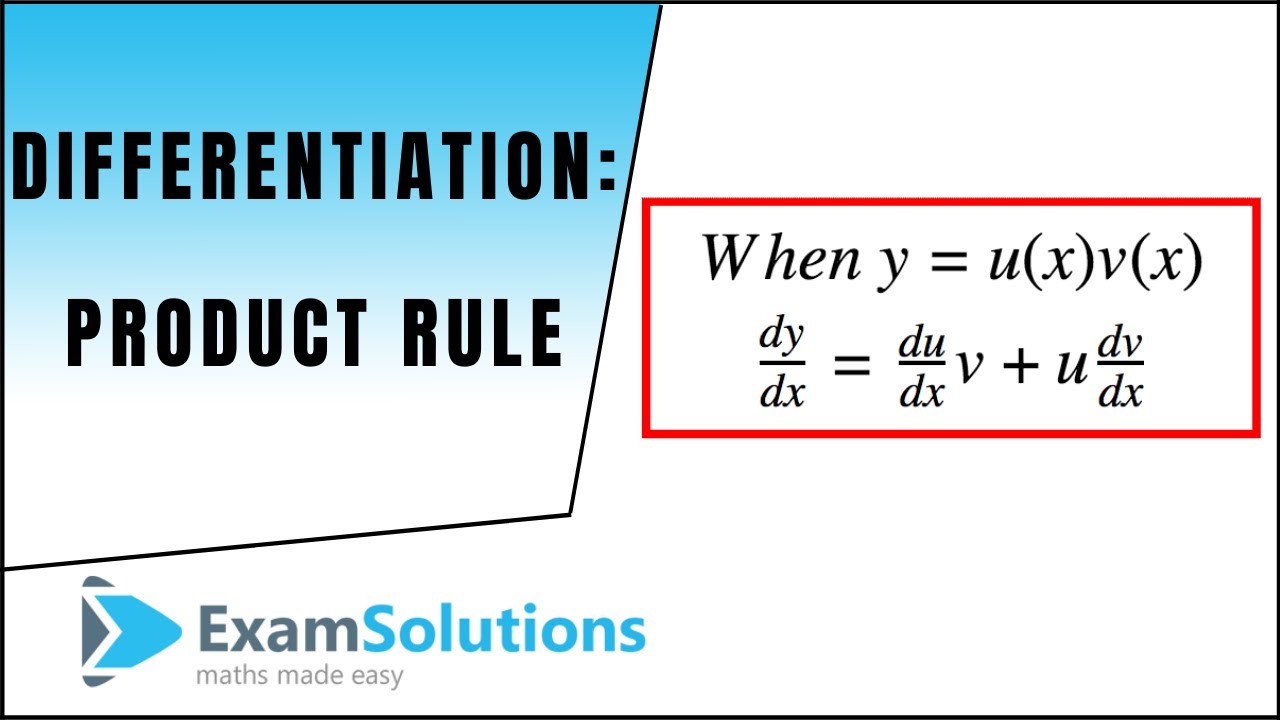
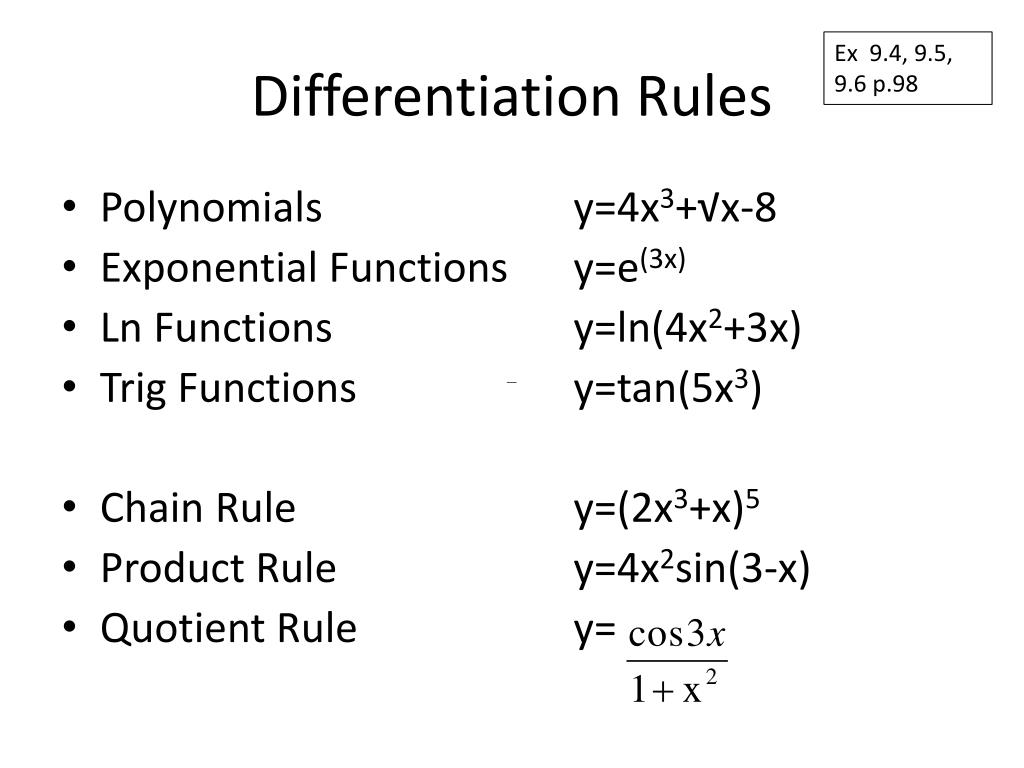
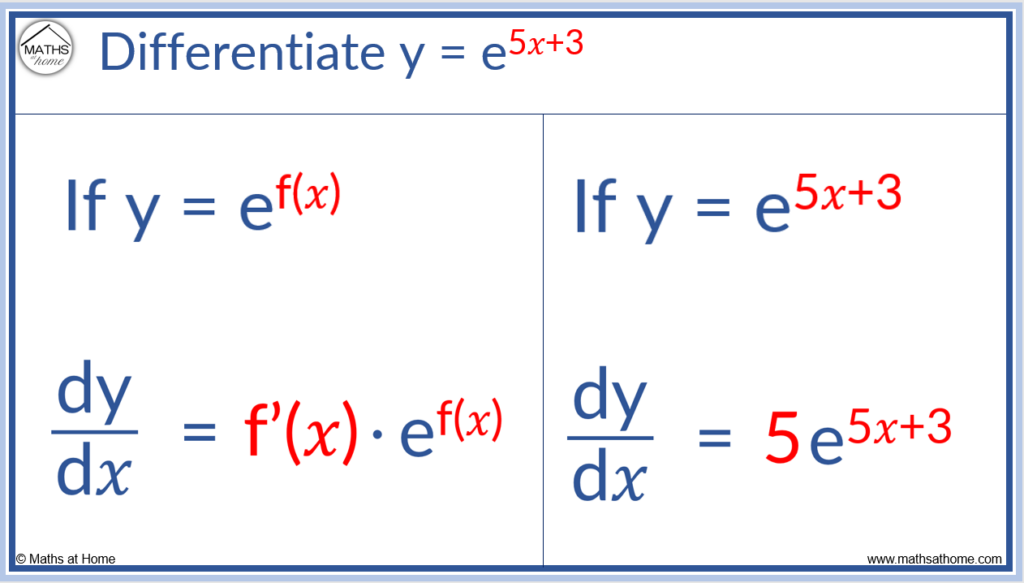
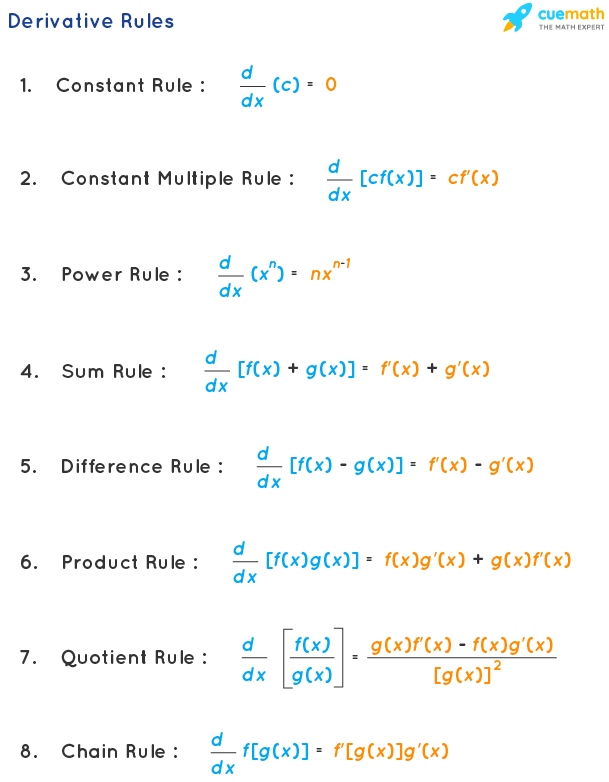
Differentiation Rules For E - 2x = (eln2)x = exln2. Next, we apply the chain rule. When the exponential expression is something other than simply x, we apply the. We first convert into base e e as follows: 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }.
2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. Next, we apply the chain rule. 2x = (eln2)x = exln2. We first convert into base e e as follows: When the exponential expression is something other than simply x, we apply the.
When the exponential expression is something other than simply x, we apply the. 2x = (eln2)x = exln2. Next, we apply the chain rule. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. We first convert into base e e as follows:
Differentiation Maths Rules
2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. Next, we apply the chain rule. We first convert into base e e as follows: When the exponential expression is something other than simply x, we apply the.
Basic Differentiation Rules Download Free PDF Combinatorics
We first convert into base e e as follows: 2x = (eln2)x = exln2. When the exponential expression is something other than simply x, we apply the. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. Next, we apply the chain rule.
PPT Differentiation Rules PowerPoint Presentation, free download ID
Next, we apply the chain rule. When the exponential expression is something other than simply x, we apply the. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. 2x = (eln2)x = exln2. We first convert into base e e as follows:
Differentiation Rules PDF
Next, we apply the chain rule. We first convert into base e e as follows: When the exponential expression is something other than simply x, we apply the. 2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }.
Rules Of Differentiation 2023
When the exponential expression is something other than simply x, we apply the. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. 2x = (eln2)x = exln2. We first convert into base e e as follows: Next, we apply the chain rule.
The Chain Rule Made Easy Examples and Solutions
2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. 2x = (eln2)x = exln2. Next, we apply the chain rule. We first convert into base e e as follows: When the exponential expression is something other than simply x, we apply the.
Differentiation Maths Rules
Next, we apply the chain rule. When the exponential expression is something other than simply x, we apply the. We first convert into base e e as follows: 2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }.
Differentiation Rules Pdf
2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. Next, we apply the chain rule. When the exponential expression is something other than simply x, we apply the. We first convert into base e e as follows:
SOLUTION Basic rules of differentiation Studypool
We first convert into base e e as follows: Next, we apply the chain rule. 2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. When the exponential expression is something other than simply x, we apply the.
Methods and Applications of Differentiation A Summary Guide
2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }. 2x = (eln2)x = exln2. Next, we apply the chain rule. When the exponential expression is something other than simply x, we apply the. We first convert into base e e as follows:
We First Convert Into Base E E As Follows:
When the exponential expression is something other than simply x, we apply the. Next, we apply the chain rule. 2x = (eln2)x = exln2. 2^x = \left ( e^ { \ln 2 } \right) ^ x = e^ { x \ln 2 }.