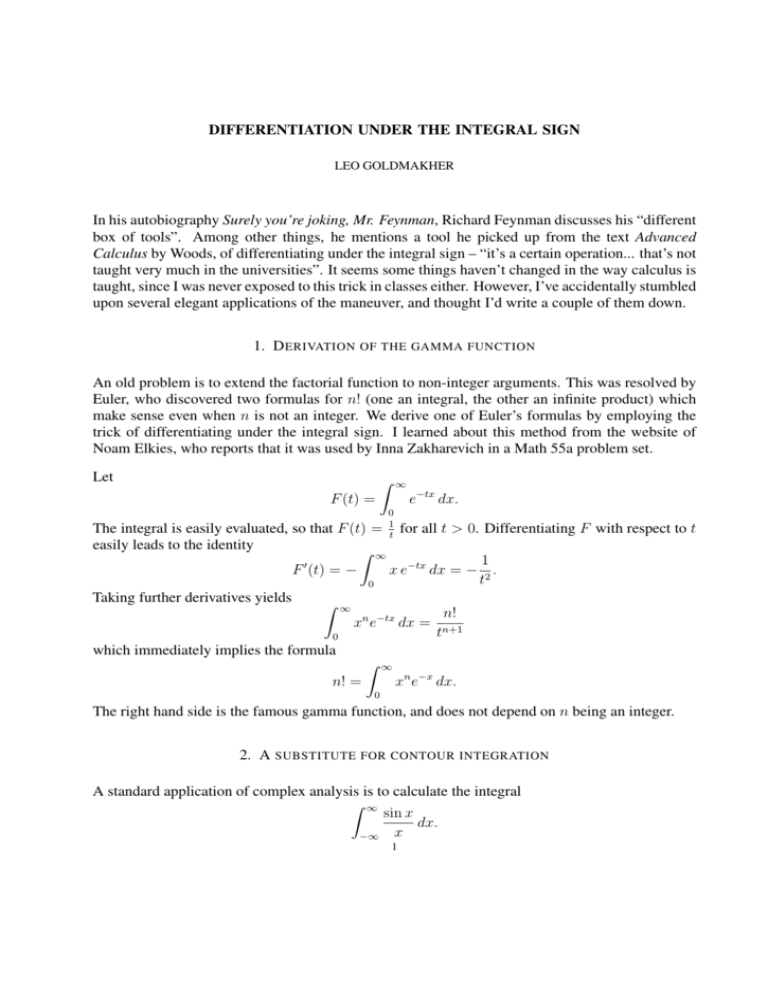
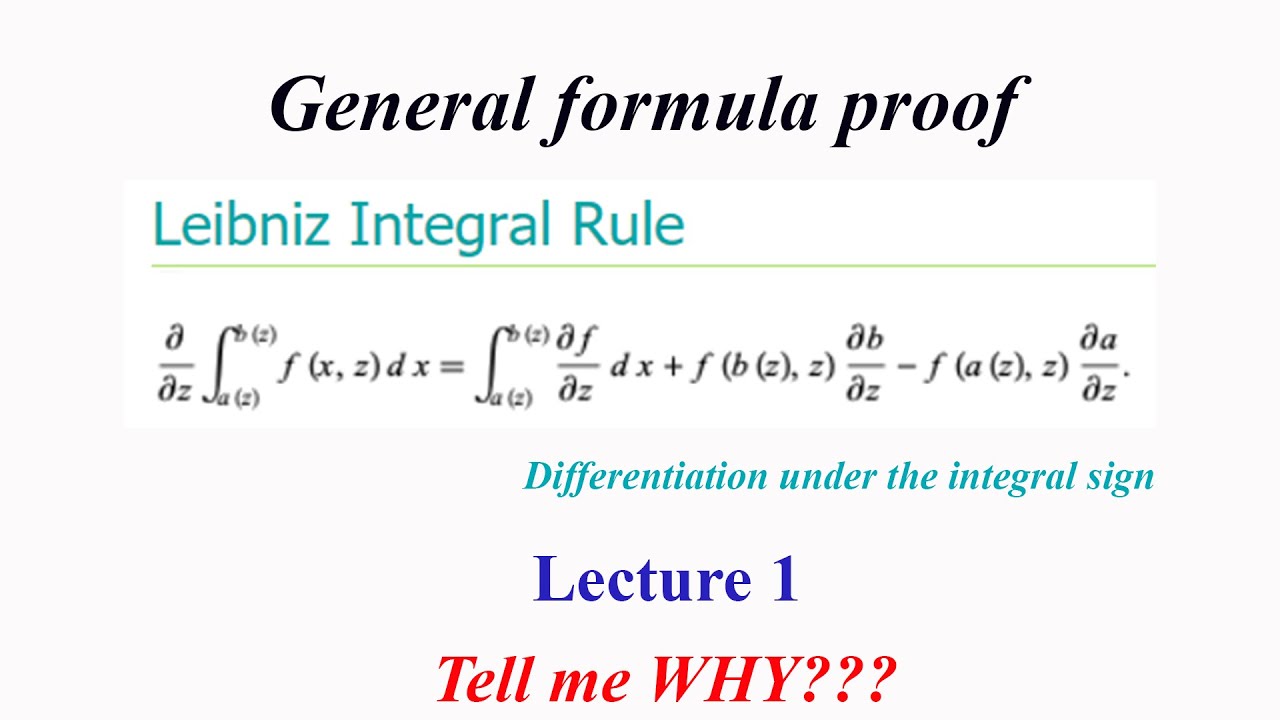
Differentiating Under The Integral Sign - To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool in calculus that. Under fairly loose conditions on the. Where in the first integral x ≥ s and |x−s| =. Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals.
Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals. Find the solution of the following integral equation: Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool in calculus that. Where in the first integral x ≥ s and |x−s| =. Under fairly loose conditions on the. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1.
Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool in calculus that. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. Under fairly loose conditions on the. Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. Where in the first integral x ≥ s and |x−s| =.
Differentiation Under The Integral Sign 2 PDF Integral Derivative
Find the solution of the following integral equation: This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Where in the first integral x ≥ s and |x−s| =. Under fairly loose conditions on the. Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1.
SOLUTION Differentiation under the integral sign Studypool
This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. Where in the first integral x ≥ s and |x−s| =. Leibnitz's theorem, also known as.
Differentiating Under The Integral Sign Download Free PDF Integral
Where in the first integral x ≥ s and |x−s| =. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Under fairly loose conditions on the. Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1.
روش افتراق تحت علامت انتگرال وبلاگ کتابخانه دیجیتال بلیان
Find the solution of the following integral equation: Where in the first integral x ≥ s and |x−s| =. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. Under fairly loose conditions on the.
Differentiating under the integral sign
Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. Under fairly loose conditions on the. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Where in the first integral x ≥ s and |x−s| =.
Integral Sign
Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals. Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. Leibnitz's theorem, also known as the leibniz rule.
Differentiation Under Integral Sign Part 1 YouTube
This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a.
[Solved] Please help me solve this differentiating under the integral
Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool in calculus that. To differentiate the integral with respect to x, we use the leibniz rule, also known as the leibniz integral rule or the differentiation under the. Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds =.
Differentiating Under The Integral Sign PDF Integral Derivative
This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals. Find the solution of the following integral equation: Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool.
SOLUTION Differentiation under integral sign Studypool
Find the solution of the following integral equation: Φ(x) + |x − s|φ(s)ds = x, −1 ≤ x ≤ 1. Where in the first integral x ≥ s and |x−s| =. This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. To differentiate the integral with respect to x, we use.
To Differentiate The Integral With Respect To X, We Use The Leibniz Rule, Also Known As The Leibniz Integral Rule Or The Differentiation Under The.
This operation, called differentiating under the integral sign, was first used by leibniz, one of the inventors of calculus. Where in the first integral x ≥ s and |x−s| =. Leibnitz's theorem, also known as the leibniz rule for differentiation under the integral sign, is a powerful tool in calculus that. Differentiation under the integral sign is an operation in calculus used to evaluate certain integrals.
Φ(X) + |X − S|Φ(S)Ds = X, −1 ≤ X ≤ 1.
Find the solution of the following integral equation: Under fairly loose conditions on the.