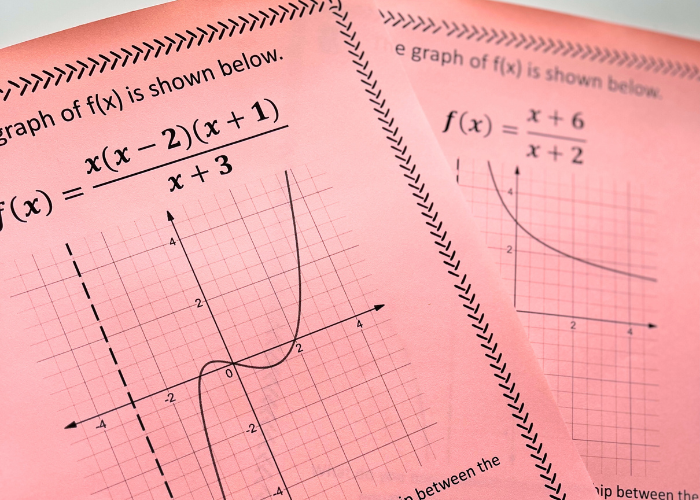
Differentiating Rational Functions - Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. The derivative of a rational function measures how the function changes at any given point. Rational functions are an important and useful class of functions, but there are others. We actually get most useful functions by starting. This function (and any other rational function) can be differentiated. It is seen as crucial knowledge in. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them.
You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. The derivative of a rational function measures how the function changes at any given point. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. We actually get most useful functions by starting. This function (and any other rational function) can be differentiated. It is seen as crucial knowledge in. Rational functions are an important and useful class of functions, but there are others. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules.
We actually get most useful functions by starting. The derivative of a rational function measures how the function changes at any given point. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. This function (and any other rational function) can be differentiated. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. Rational functions are an important and useful class of functions, but there are others. It is seen as crucial knowledge in.
Rational Functions Studocu
It is seen as crucial knowledge in. This function (and any other rational function) can be differentiated. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. The derivative of a rational function measures how the.
Rational Functions PDF Asymptote Equations
The derivative of a rational function measures how the function changes at any given point. We actually get most useful functions by starting. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. You should practice finding the.
Rational Functions Archives Mathy Mrs. J
We actually get most useful functions by starting. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. This function (and any other rational function) can be differentiated. The derivative of a rational.
Rational Functions Math Steps, Examples & Questions
Rational functions are an important and useful class of functions, but there are others. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. We actually get most useful functions by starting. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. It is seen as.
Solved It is a common misconception when differentiating
Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. The derivative of a rational function measures how the function changes at any given point. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. We actually get most useful functions by starting. It is seen as crucial.
Rational Functions Unit Bundle Clark Creative Education
Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. Rational functions are an important.
RationalFunctionsEquationsandInequalities.pptx Free Download
This function (and any other rational function) can be differentiated. Rational functions are an important and useful class of functions, but there are others. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel.
Rational Functions Math Steps, Examples & Questions
Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. The derivative of a rational function measures how the function changes at any given point. We actually get most useful functions by starting. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and. You should practice.
Graphing rational functions PPT
This function (and any other rational function) can be differentiated. It is seen as crucial knowledge in. We actually get most useful functions by starting. The derivative of a rational function measures how the function changes at any given point. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules.
10 Surprising Reallife Examples Of Rational Functions Number Dyslexia
This function (and any other rational function) can be differentiated. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. We actually get most useful functions by starting. You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. Remember that a rational function.
We Actually Get Most Useful Functions By Starting.
Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. It is seen as crucial knowledge in. Differentiating rational functions, with discussion and proofs of the constant, sum, product, quotient, and power rules. This function (and any other rational function) can be differentiated.
The Derivative Of A Rational Function Measures How The Function Changes At Any Given Point.
You should practice finding the derivatives of polynomials and of rational functions using these rules until you feel comfortable with them. Rational functions are an important and useful class of functions, but there are others. Remember that a rational function \(h(x)\) can be expressed in such a way that \(h(x)=\frac{f(x)}{g(x)},\) where \(f(x)\) and.