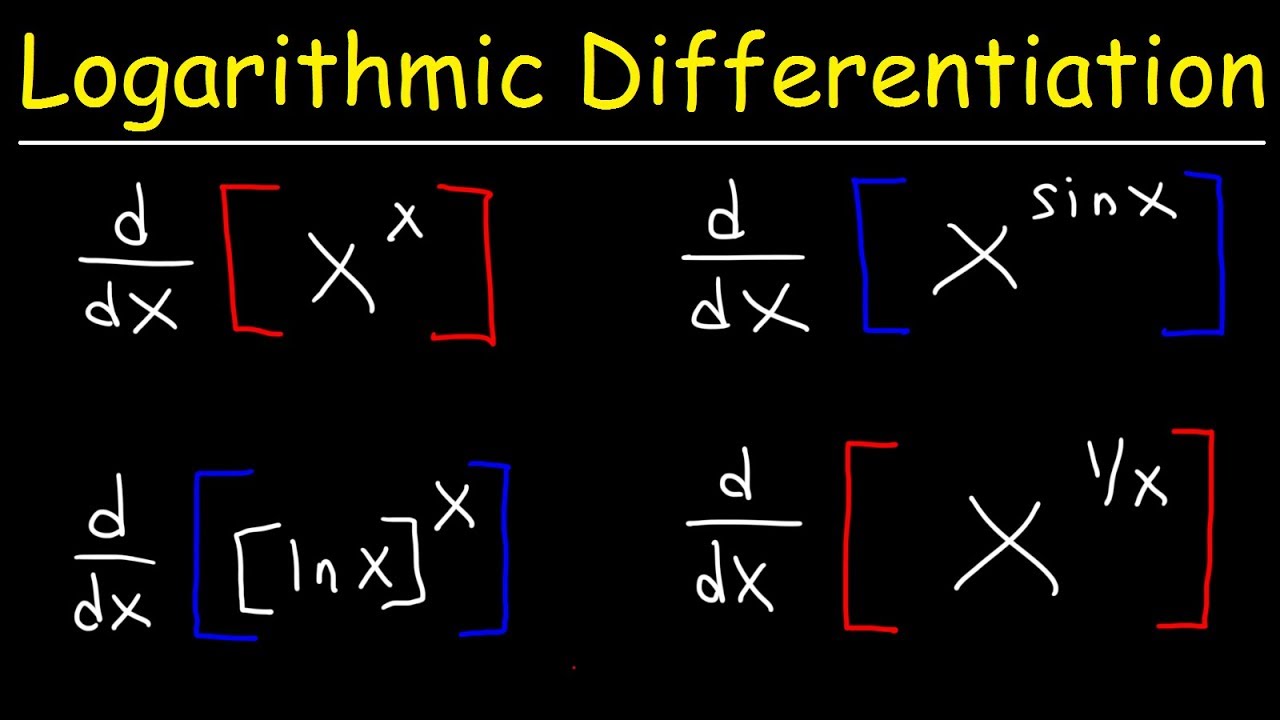
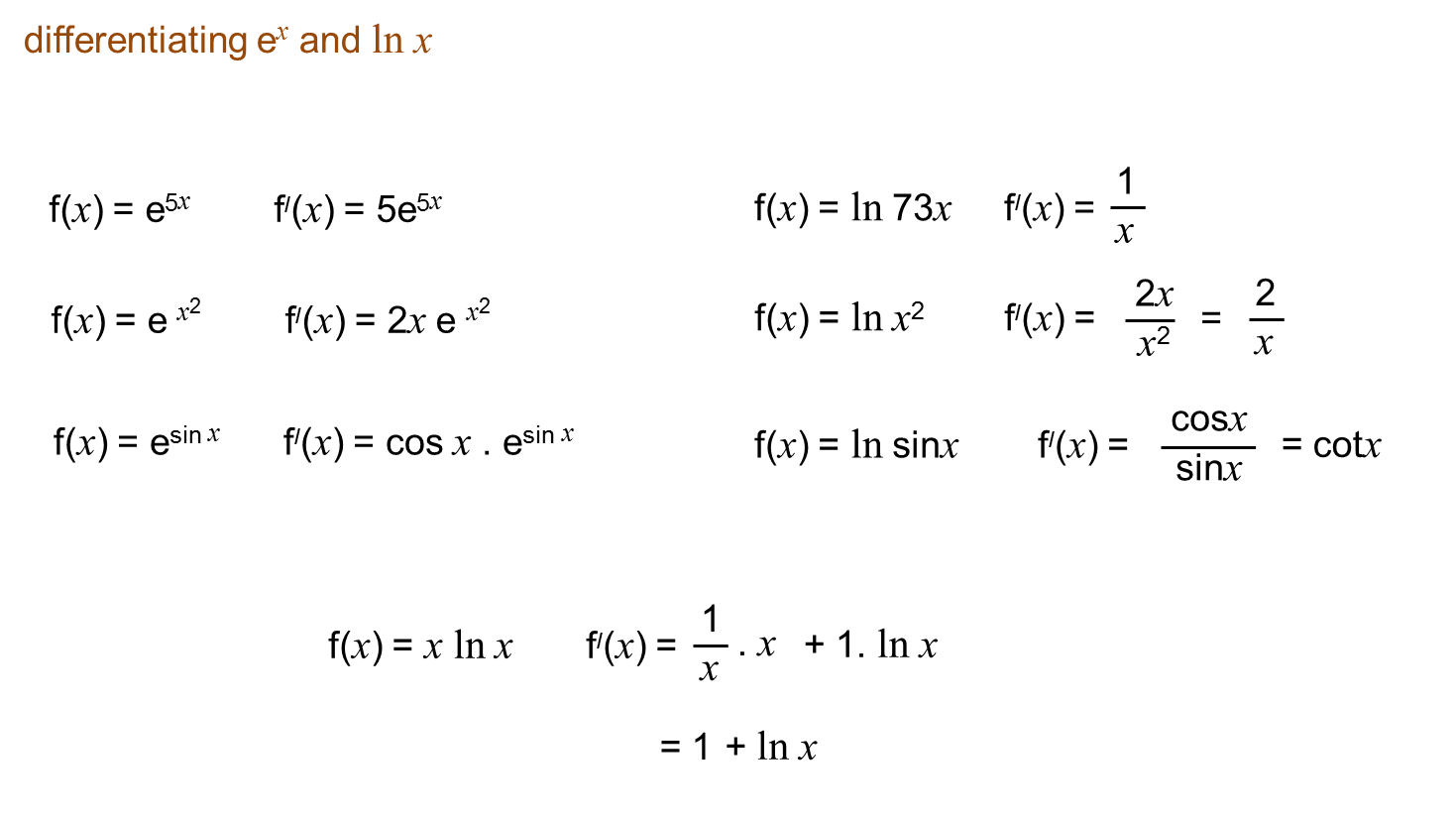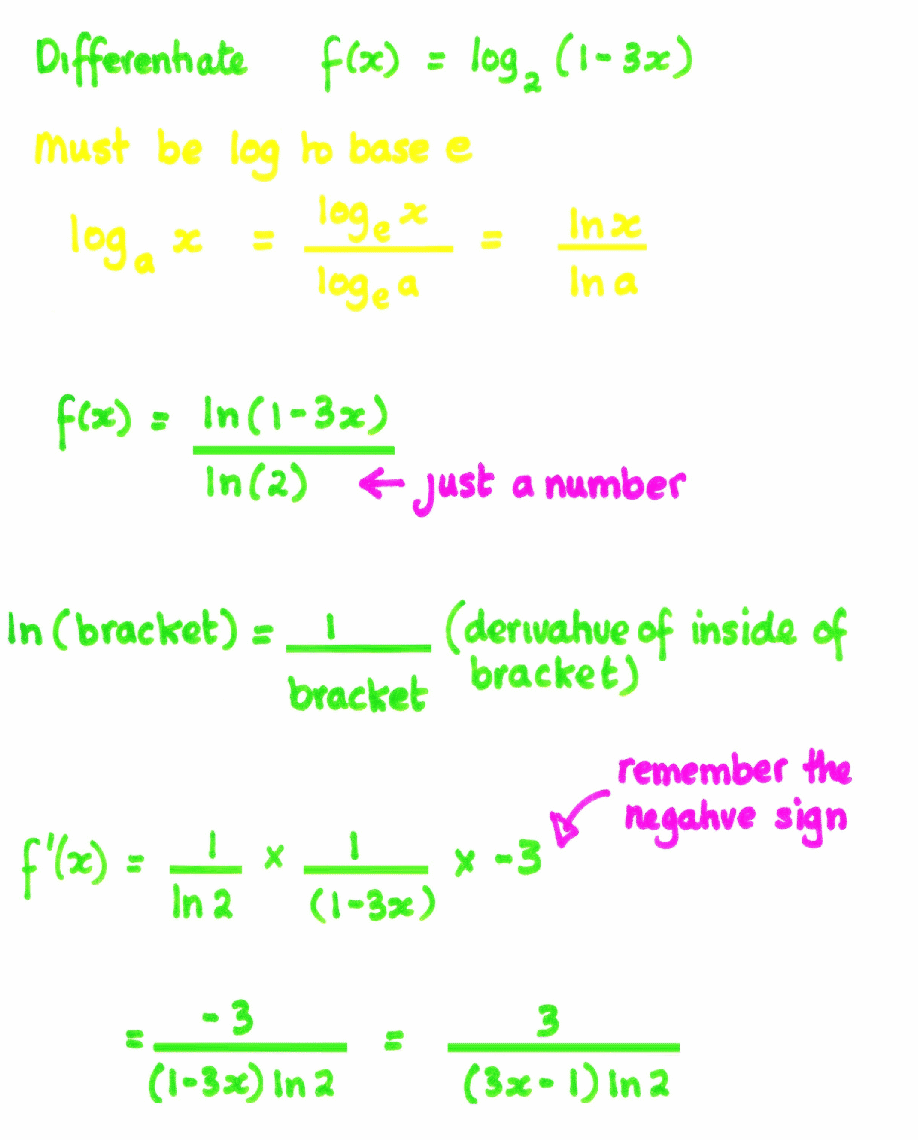Differentiating Logs - Derivatives of logarithmic functions are mainly based on the chain rule. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with.
However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Derivatives of logarithmic functions are mainly based on the chain rule. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with.
CALCULUS Differentiating Natural Logs YouTube
Derivatives of logarithmic functions are mainly based on the chain rule. However, we can generalize it for any differentiable function with. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
12X1 T01 02 differentiating logs
However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Exponential Functions And Logs
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule.
Differentiating with Logs EX1 YouTube
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule.
12X1 T01 02 differentiating logs
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule.
Introduction to Logarithmic Differentiation YouTube
However, we can generalize it for any differentiable function with. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Derivatives of logarithmic functions are mainly based on the chain rule.
Differentiating Natural log Functions 2 YouTube
However, we can generalize it for any differentiable function with. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Derivatives of logarithmic functions are mainly based on the chain rule.
core pure 3 notes differentiating natural logarithms and exponentials
Derivatives of logarithmic functions are mainly based on the chain rule. However, we can generalize it for any differentiable function with. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
12 x1 t01 02 differentiating logs (2013) PPT
However, we can generalize it for any differentiable function with. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. Derivatives of logarithmic functions are mainly based on the chain rule.
Using Logs to help differentiate
Derivatives of logarithmic functions are mainly based on the chain rule. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with.
Derivatives Of Logarithmic Functions Are Mainly Based On The Chain Rule.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. However, we can generalize it for any differentiable function with.