Differential Equations Initial Conditions - For a second order differential equation we have three possible types of boundary conditions: With these two initial conditions and the general solution to the differential equation, we can find. In this unit our differential equations will always have initial conditions at t = 0. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. Pde’s are usually specified through a set of boundary or initial conditions.
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find.
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0.
Differential Equations
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: Pde’s are usually specified through a set of boundary or initial conditions. In this unit.
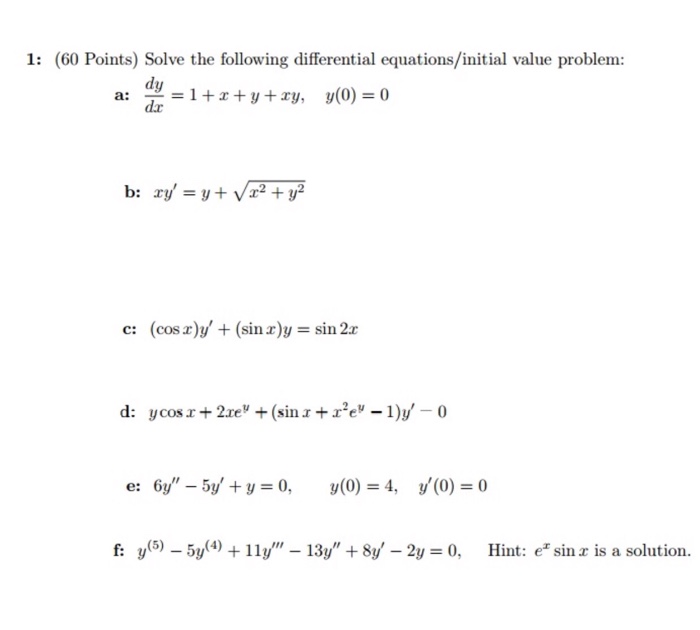
Solved Solve the following differential equations/initial
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. For a second order differential equation we have three possible types of boundary conditions: With these two initial.
Differential Equations Solver
With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Solve a differential equation analytically by using the dsolve function, with or without initial conditions..
Exact Differential Equations
For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. With these two initial conditions and the general solution to the differential equation, we can find. Solve a differential equation analytically by using the dsolve function, with or without initial conditions..
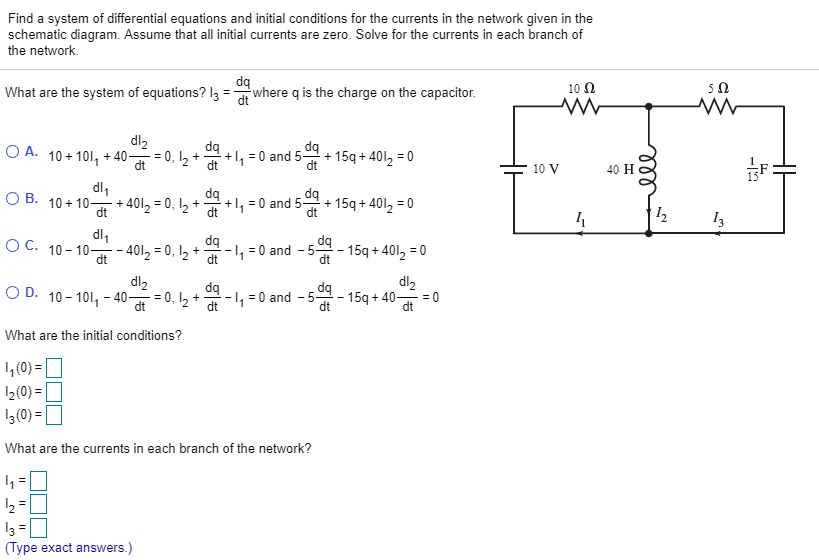
Solved Find a system of differential equations and initial
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial.
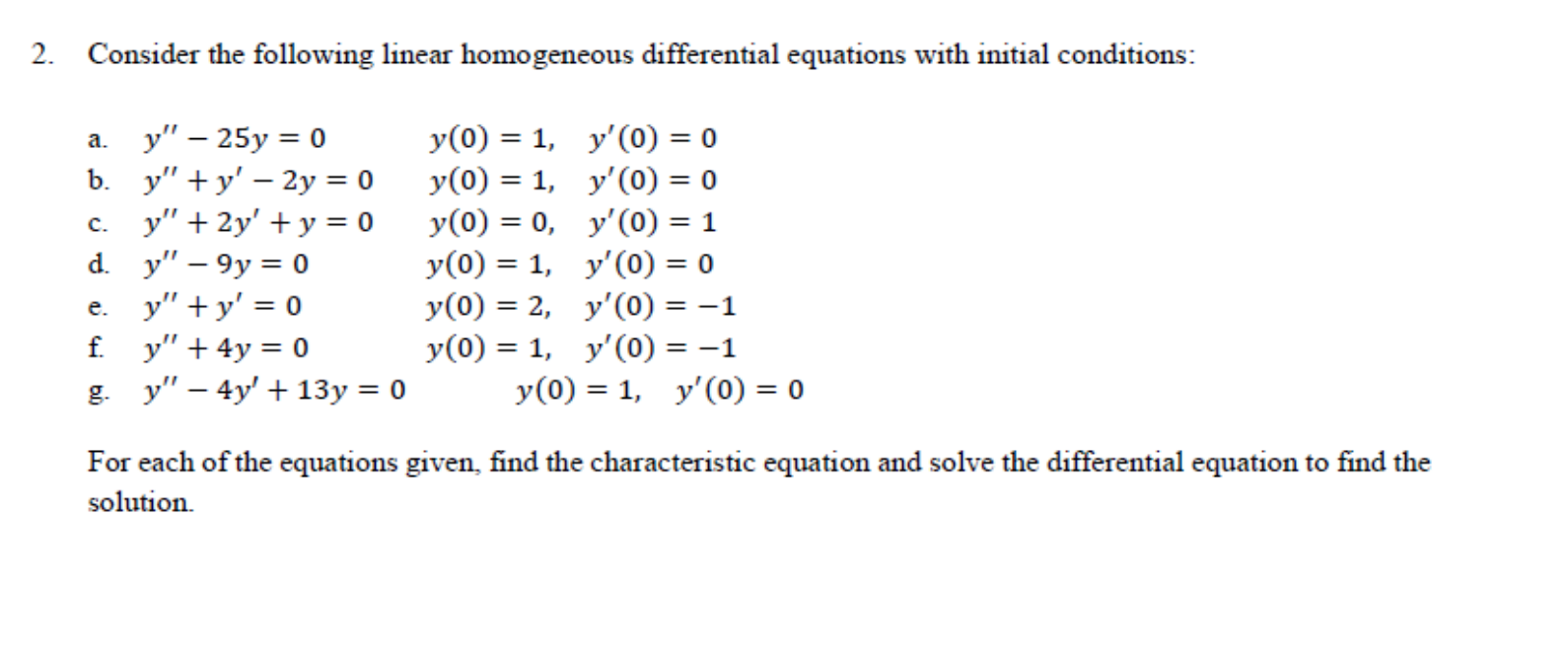
Solved Consider the following linear homogeneous
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit.
(PDF) Numerical Methods for Ordinary Differential Equations Initial
For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. Solve a differential.
Solved Solve each one of the following differential
With these two initial conditions and the general solution to the differential equation, we can find. Pde’s are usually specified through a set of boundary or initial conditions. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. For a.
Solved Consider the system of differential equations with
For a second order differential equation we have three possible types of boundary conditions: Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. With these two initial conditions and the general solution to the differential equation, we can find..
[Solved] differential equation (1 point) A 10 kilogram object suspended
Pde’s are usually specified through a set of boundary or initial conditions. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. With these two initial conditions and the general solution to the differential equation, we can find. For a.
Solve A Differential Equation Analytically By Using The Dsolve Function, With Or Without Initial Conditions.
In this unit our differential equations will always have initial conditions at t = 0. For a second order differential equation we have three possible types of boundary conditions: Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find.