6Th Order Differential Equation - $x$, $y$, $z$, $v_x$, $v_y$, and. This paper aims to investigate the existence and uniqueness of solutions for a sixth. I want to solve one system of ode problem with numerical methods. I've been able to formulate the characteristic polynomial: = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. An object moving in three dimensions is described by six variables:
= 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. $x$, $y$, $z$, $v_x$, $v_y$, and. This paper aims to investigate the existence and uniqueness of solutions for a sixth. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. An object moving in three dimensions is described by six variables: I've been able to formulate the characteristic polynomial: I want to solve one system of ode problem with numerical methods.
An object moving in three dimensions is described by six variables: $x$, $y$, $z$, $v_x$, $v_y$, and. I've been able to formulate the characteristic polynomial: I want to solve one system of ode problem with numerical methods. This paper aims to investigate the existence and uniqueness of solutions for a sixth. = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer.
4.second Order Differential Equation Examples PDF Ordinary
= 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. I've been able to formulate the characteristic polynomial: This paper aims to investigate the existence and uniqueness of solutions for a sixth..
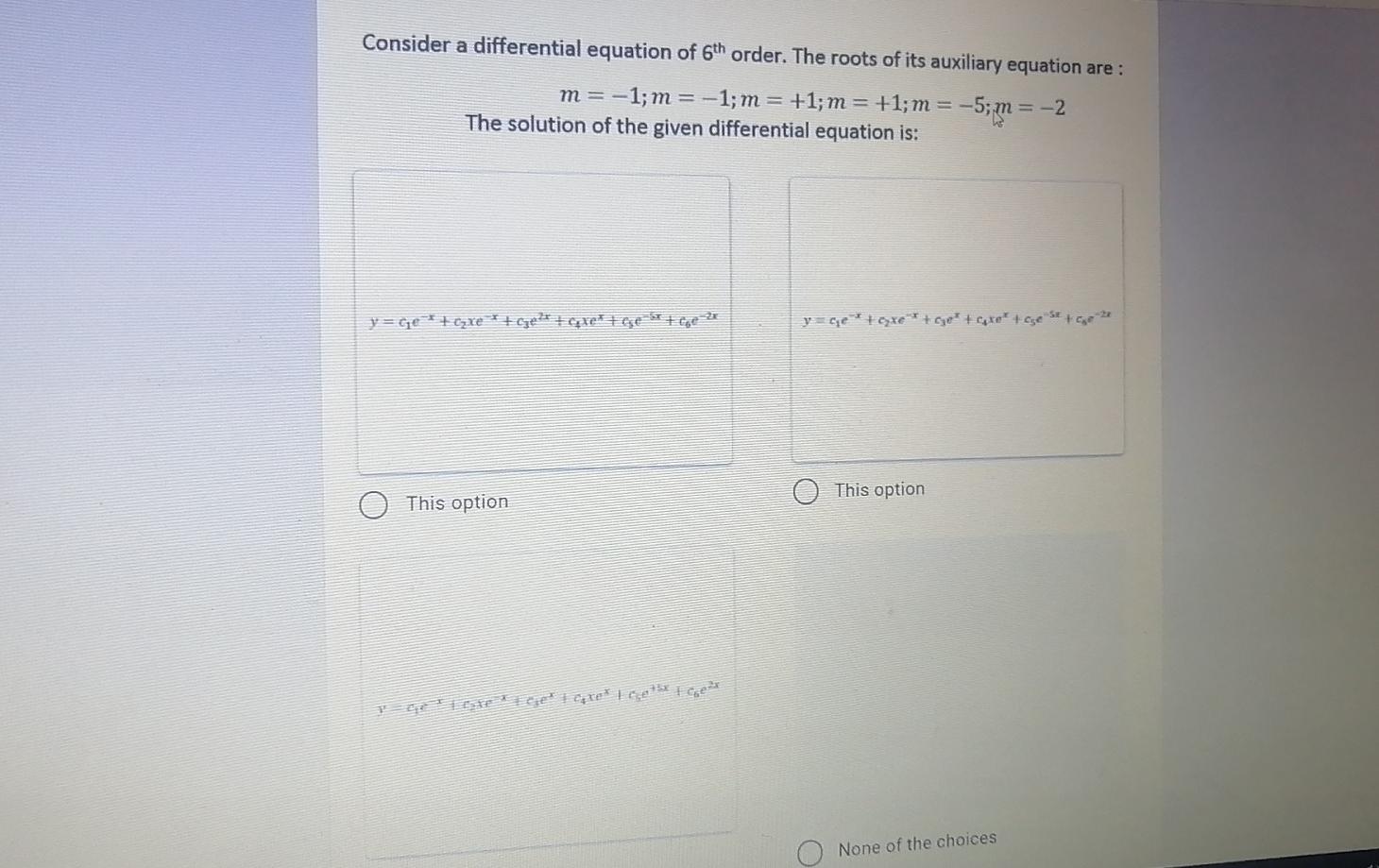
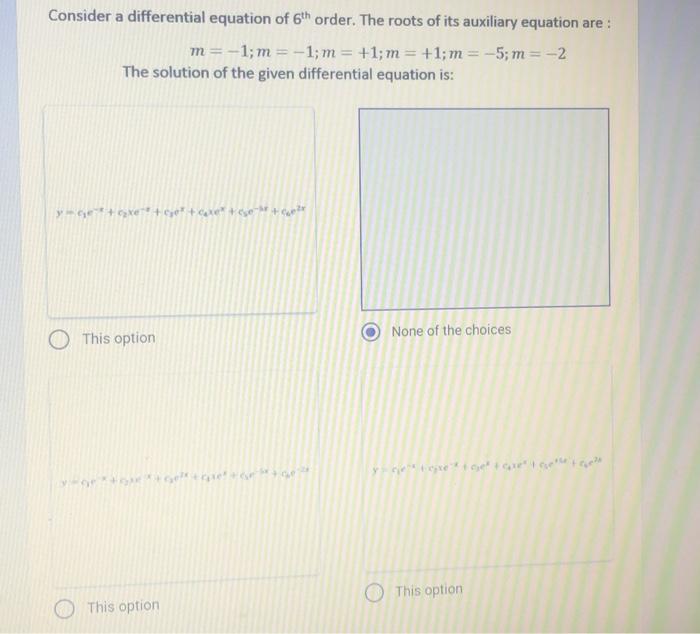
Solved Consider a differential equation of 6th order. The
I've been able to formulate the characteristic polynomial: An object moving in three dimensions is described by six variables: This paper aims to investigate the existence and uniqueness of solutions for a sixth. = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. I want to solve one system of ode problem.
[Solved] DIFFERENTIAL EQUATION APPLICATION OF FIRSTORDER DIFFERENTIAL
$x$, $y$, $z$, $v_x$, $v_y$, and. An object moving in three dimensions is described by six variables: This paper aims to investigate the existence and uniqueness of solutions for a sixth. I've been able to formulate the characteristic polynomial: I want to solve one system of ode problem with numerical methods.
SOLVED Question 2 (1 point) Saved Classify the differential equation
I want to solve one system of ode problem with numerical methods. I've been able to formulate the characteristic polynomial: This paper aims to investigate the existence and uniqueness of solutions for a sixth. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. $x$, $y$, $z$, $v_x$, $v_y$, and.
SOLVED Suppose that a fourthorder differential equation has a
I want to solve one system of ode problem with numerical methods. = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. An object moving in three dimensions is described by six variables: I've been able to formulate the characteristic polynomial: This paper aims to investigate the existence and uniqueness of solutions.
Solved Consider a differential equation of 6th order. The
It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. $x$, $y$, $z$, $v_x$, $v_y$, and. I've been able to formulate the characteristic polynomial: An object moving in three dimensions is described by six variables: This paper aims to investigate the existence and uniqueness of solutions for a sixth.
Order and Degree of Differential Equation Concepts, Videos & Examples
An object moving in three dimensions is described by six variables: $x$, $y$, $z$, $v_x$, $v_y$, and. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. This paper aims to investigate the existence and uniqueness of solutions for a sixth. I've been able to formulate the characteristic polynomial:
SOLUTION Second order differential equation 1 Studypool
An object moving in three dimensions is described by six variables: This paper aims to investigate the existence and uniqueness of solutions for a sixth. I want to solve one system of ode problem with numerical methods. $x$, $y$, $z$, $v_x$, $v_y$, and. It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms.
6th Differential Equation PDF
This paper aims to investigate the existence and uniqueness of solutions for a sixth. An object moving in three dimensions is described by six variables: $x$, $y$, $z$, $v_x$, $v_y$, and. = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. I want to solve one system of ode problem with numerical.
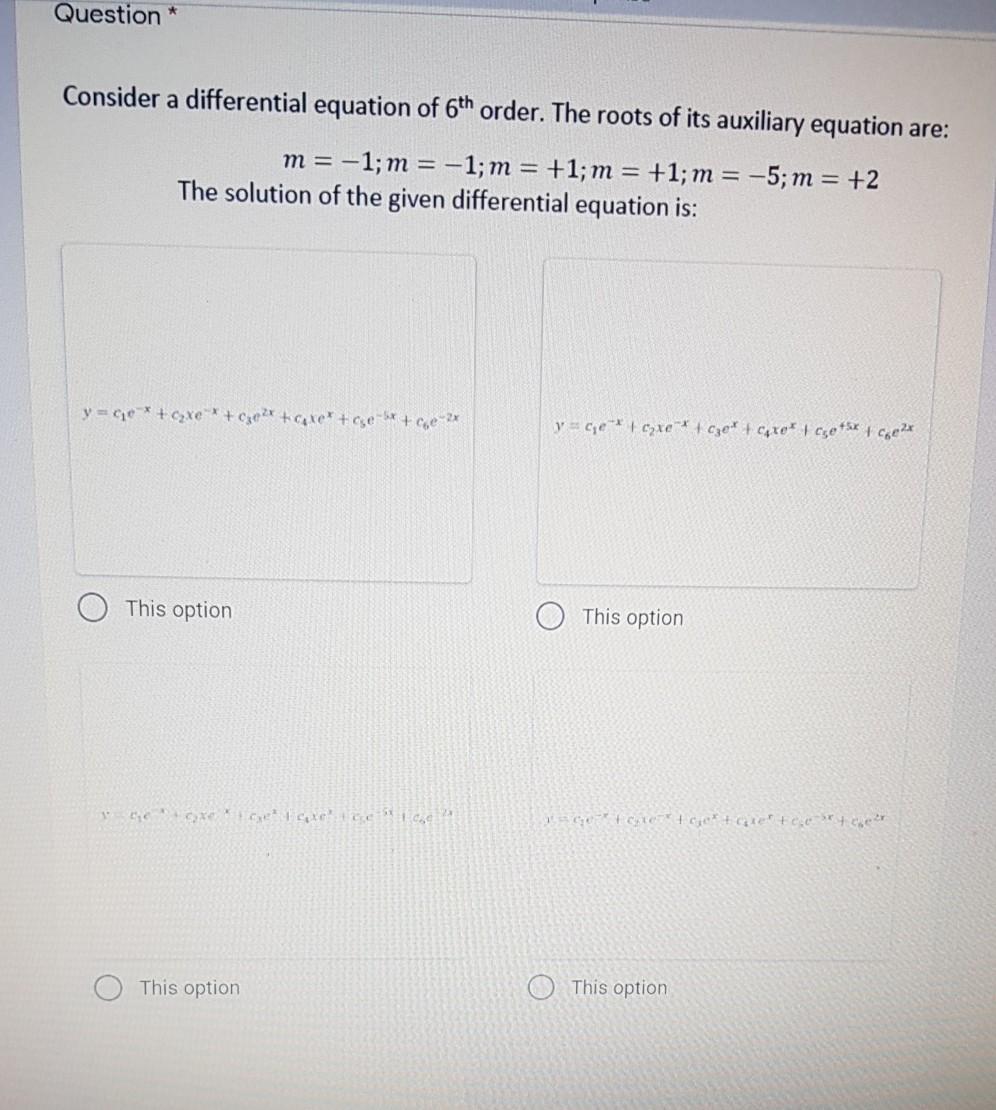
Solved Question * Consider a differential equation of 6th
It's the multiplicity that necessitates the factor of x x in the 2nd, 5th, and 6th terms in the answer. An object moving in three dimensions is described by six variables: $x$, $y$, $z$, $v_x$, $v_y$, and. = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. I want to solve one.
It's The Multiplicity That Necessitates The Factor Of X X In The 2Nd, 5Th, And 6Th Terms In The Answer.
An object moving in three dimensions is described by six variables: = 0 gives the standard 4th order central di erence scheme, = 1=10 gives the classical pad e. $x$, $y$, $z$, $v_x$, $v_y$, and. I've been able to formulate the characteristic polynomial:
This Paper Aims To Investigate The Existence And Uniqueness Of Solutions For A Sixth.
I want to solve one system of ode problem with numerical methods.